Antiparallel
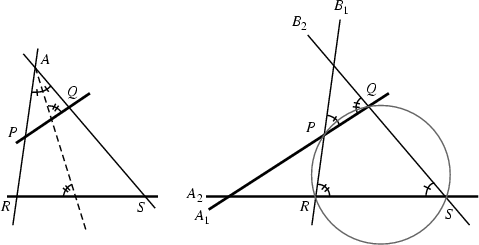
Two lines 
and 
are said to be antiparallel with respect to the sides of an Angle 
if they make the sameangle in the opposite senses with the Bisector of that angle. If and are antiparallel withrespect to 
and 
, then the latter are also antiparallel with respect to the former. Furthermore, if and areantiparallel, then the points 
, 
, 
, and 
are Concyclic (Johnson 1929, p. 172; Honsberger 1995, pp. 87-88).
References
Honsberger, R. ``Parallels and Antiparallels.'' §9.1 in Episodes in Nineteenth and Twentieth Century Euclidean Geometry. Washington, DC: Math. Assoc. Amer., pp. 87-88, 1995. Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, p. 172, 1929.
- Morse Inequalities
- Morse Theory
- Morse-Thue Sequence
- Mortal
- Mortality Problem
- Morton-Franks-Williams Inequality
- Mosaic
- Moser
- Moser's Circle Problem
- Moss's Egg
- Motzkin Number
- Moufang Plane
- Mousetrap
- Mouth
- Moving Average
- Moving Ladder Constant
- Moving Sofa Constant
- Mrs. Perkins' Quilt
- Much Greater
- Much Less
- Mu Function
- Muirhead's Theorem
- Muller's Method
- Mulliken Symbols
- Multiamicable Numbers