“Arbelos”的概念、定义、习题解题方法及翻译-数学辞典

单词

Arbelos

释义

Arbelos

The term ``arbelos'' means Shoemaker's Knife in Greek, and this term is applied to the shaded Area in the abovefigure which resembles the blade of a knife used by ancient cobblers (Gardner 1979). Archimedes himself is believedto have been the first mathematician to study the mathematical properties of this figure. The position of the central notch isarbitrary and can be located anywhere along the Diameter.

The arbelos satisfies a number of unexpected identities (Gardner 1979).

1. Call the radii of the left and right Semicircles

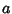and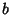

, respectively, with

. Thenthe arc length along the bottom of the arbelos is

so the arc lengths along the top and bottom of the arbelos are the same.

: 2. Draw the Perpendicular 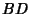from the tangent of the two Semicircles to the edge of thelarge Circle. Then the Area of the arbelos is the same as the Area of the Circle withDiameter .
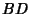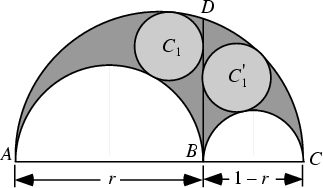
: 3. The Circles 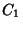and 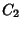inscribed on each half of 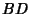on the arbelos (called Archimedes'Circles) each have Diameter . Furthermore, the smallest Circumcircle of these two circles has anarea equal to that of the arbelos.
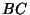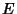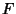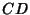: 4. The line tangent to the semicircles and contains the point and which lie on the lines and, respectively. Furthermore, 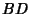and 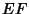bisect each other, and the points 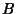, , 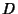, 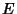and 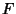are Concyclic.

: 5. In addition to the Archimedes' Circles 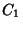and 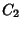in the arbelos figure, there is a third circle 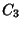called the Bankoff Circle which is congruent to these two.

6. Construct a chain of Tangent Circles starting with the Circle Tangent to thetwo small ones and large one. The centers of the Circles lie on an Ellipse, and theDiameter of the

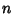th Circle

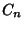is (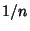

)th Perpendicular distance to the base of thePappus,

whoreferred to it as an ancient theorem (Hood 1961, Cadwell 1966, Gardner 1979, Bankoff 1981). If

, then the radiusof the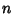

th circle in the Pappus Chain is

This general result simplifies to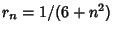

for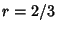

(Gardner 1979). Further special cases when

are considered by Gaba (1940).

7. If

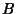divides

in the Golden Ratio

, then the circles in the chain satisfy a number of otherspecial properties (Bankoff 1955).

See also Archimedes' Circles, Bankoff Circle, Coxeter's Loxodromic Sequence of Tangent Circles, Golden Ratio,Inversion, Pappus Chain, Steiner Chain

References

Bankoff, L. ``The Fibonacci Arbelos.'' Scripta Math. 20, 218, 1954.

Bankoff, L. ``The Golden Arbelos.'' Scripta Math. 21, 70-76, 1955.

Bankoff, L. ``Are the Twin Circles of Archimedes Really Twins?'' Math. Mag. 47, 214-218, 1974.

Bankoff, L. ``How Did Pappus Do It?'' In The Mathematical Gardner (Ed. D. Klarner). Boston, MA: Prindle, Weber, and Schmidt, pp. 112-118, 1981.

Bankoff, L. ``The Marvelous Arbelos.'' In The Lighter Side of Mathematics (Ed. R. K. Guy and R. E. Woodrow). Washington, DC: Math. Assoc. Amer., 1994.

Cadwell, J. H. Topics in Recreational Mathematics. Cambridge, England: Cambridge University Press, 1966.

Gaba, M. G. ``On a Generalization of the Arbelos.'' Amer. Math. Monthly 47, 19-24, 1940.

Gardner, M. ``Mathematical Games: The Diverse Pleasures of Circles that Are Tangent to One Another.'' Sci. Amer. 240, 18-28, Jan. 1979.

Heath, T. L. The Works of Archimedes with the Method of Archimedes. New York: Dover, 1953.

Hood, R. T. ``A Chain of Circles.'' Math. Teacher 54, 134-137, 1961.

Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 116-117, 1929.

Ogilvy, C. S. Excursions in Geometry. New York: Dover, pp. 54-55, 1990.

随便看

数学辞典收录了8975条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。