| 释义 |
Bell NumberThe number of ways a Set of 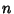 elements can be Partitioned into nonemptySubsets is called a Bell Number and is denoted elements can be Partitioned into nonemptySubsets is called a Bell Number and is denoted  . For example, there are five ways thenumbers . For example, there are five ways thenumbers  1, 2, 3 1, 2, 3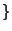 can be partitioned: can be partitioned: 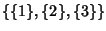 , ,  , ,  , ,  , and , and  , so , so 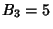 . .  and the first few Bellnumbers for and the first few Bellnumbers for 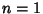 , 2, ... are 1, 2, 5, 15, 52, 203, 877, 4140, 21147, 115975, ... (Sloane's A000110). Bell numbers areclosely related to Catalan Numbers. , 2, ... are 1, 2, 5, 15, 52, 203, 877, 4140, 21147, 115975, ... (Sloane's A000110). Bell numbers areclosely related to Catalan Numbers.
The diagram below shows the constructions giving 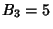 and and  , with line segments representing elements in thesame Subset and dots representing subsets containing a single element (Dickau). , with line segments representing elements in thesame Subset and dots representing subsets containing a single element (Dickau). The Integers  can be defined by the sum can be defined by the sum
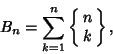 | (1) |
 is a Stirling Number of the Second Kind, or by the generating function is a Stirling Number of the Second Kind, or by the generating function
 | (2) |
 | (3) |
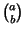 is a Binomial Coefficient, or using the formula of Comtet (1974) is a Binomial Coefficient, or using the formula of Comtet (1974)
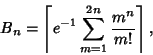 | (4) |
 denotes the Ceiling Function. denotes the Ceiling Function.
The Bell number  is also equal to is also equal to 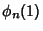 , where , where 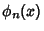 is a Bell Polynomial. Dobinski'sFormula gives the is a Bell Polynomial. Dobinski'sFormula gives the 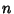 th Bell number th Bell number
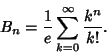 | (5) |
 | (6) |
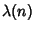 is defined implicitly by the equation is defined implicitly by the equation
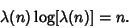 | (7) |
 | (8) |
 | (9) |
Touchard's Congruence states
 | (10) |
 is Prime. The only Prime Bell numbers for is Prime. The only Prime Bell numbers for 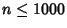 are are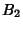 , , 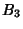 , , 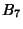 , , 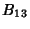 , , 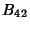 , and , and 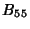 . The Bell numbers also have the curious property that . The Bell numbers also have the curious property that
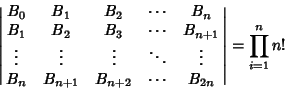 | (11) |
References
Bell, E. T. ``Exponential Numbers.'' Amer. Math. Monthly 41, 411-419, 1934.Comtet, L. Advanced Combinatorics. Dordrecht, Netherlands: Reidel, 1974. Conway, J. H. and Guy, R. K. In The Book of Numbers. New York: Springer-Verlag, pp. 91-94, 1996. de Bruijn, N. G. Asymptotic Methods in Analysis. New York: Dover, pp. 102-109, 1958. Dickau, R. M. ``Bell Number Diagrams.''http://forum.swarthmore.edu/advanced/robertd/bell.html. Gardner, M. ``The Tinkly Temple Bells.'' Ch. 2 in Fractal Music, Hypercards, and More Mathematical Recreations from Scientific American Magazine. New York: W. H. Freeman, 1992. Gould, H. W. Bell & Catalan Numbers: Research Bibliography of Two Special Number Sequences, 6th ed. Morgantown, WV: Math Monongliae, 1985. Lenard, A. In Fractal Music, Hypercards, and More Mathematical Recreations from Scientific American Magazine. (M. Gardner). New York: W. H. Freeman, pp. 35-36, 1992. Levine, J. and Dalton, R. E. ``Minimum Periods, Modulo  , of First Order Bell Exponential Integrals.'' Math. Comput. 16, 416-423, 1962. , of First Order Bell Exponential Integrals.'' Math. Comput. 16, 416-423, 1962. Lovász, L. Combinatorial Problems and Exercises, 2nd ed. Amsterdam, Netherlands: North-Holland, 1993. Pitman, J. ``Some Probabilistic Aspects of Set Partitions.'' Amer. Math. Monthly 104, 201-209, 1997. Rota, G.-C. ``The Number of Partitions of a Set.'' Amer. Math. Monthly 71, 498-504, 1964. Sloane, N. J. A. SequenceA000110/M1484in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
|
![]() elements can be Partitioned into nonemptySubsets is called a Bell Number and is denoted
elements can be Partitioned into nonemptySubsets is called a Bell Number and is denoted ![]() . For example, there are five ways thenumbers
. For example, there are five ways thenumbers ![]() 1, 2, 3
1, 2, 3![]() can be partitioned:
can be partitioned: ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , so
, so ![]() .
. ![]() and the first few Bellnumbers for
and the first few Bellnumbers for ![]() , 2, ... are 1, 2, 5, 15, 52, 203, 877, 4140, 21147, 115975, ... (Sloane's A000110). Bell numbers areclosely related to Catalan Numbers.
, 2, ... are 1, 2, 5, 15, 52, 203, 877, 4140, 21147, 115975, ... (Sloane's A000110). Bell numbers areclosely related to Catalan Numbers.![]() and
and ![]() , with line segments representing elements in thesame Subset and dots representing subsets containing a single element (Dickau).
, with line segments representing elements in thesame Subset and dots representing subsets containing a single element (Dickau).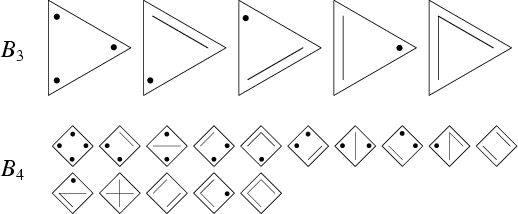
![]() can be defined by the sum
can be defined by the sum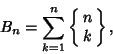
 is a Stirling Number of the Second Kind, or by the generating function
is a Stirling Number of the Second Kind, or by the generating function

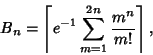
![]() is also equal to
is also equal to ![]() , where
, where ![]() is a Bell Polynomial. Dobinski'sFormula gives the
is a Bell Polynomial. Dobinski'sFormula gives the ![]() th Bell number
th Bell number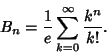

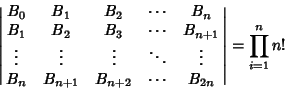
![]() , of First Order Bell Exponential Integrals.'' Math. Comput. 16, 416-423, 1962.
, of First Order Bell Exponential Integrals.'' Math. Comput. 16, 416-423, 1962.