Bézier Curve
Given a set of ![]() control points, the corresponding Bézier curve (or Bernstein-Bézier Curve) is given by
control points, the corresponding Bézier curve (or Bernstein-Bézier Curve) is given by
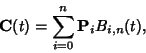
where
A ``rational'' Bézier curve is defined by
where
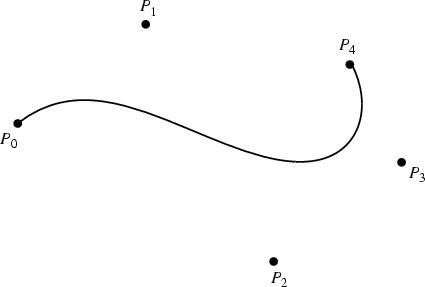
The Bézier curve always passes through the first and last control points and lies within the Convex Hull of thecontrol points. The curve is tangent to ![]() and
and ![]() at the endpoints. The``variation diminishing property'' of these curves is that no line can have more intersections with a Bézier curve thanwith the curve obtained by joining consecutive points with straight line segments. A desirable property of these curves isthat the curve can be translated and rotated by performing these operations on the control points.
at the endpoints. The``variation diminishing property'' of these curves is that no line can have more intersections with a Bézier curve thanwith the curve obtained by joining consecutive points with straight line segments. A desirable property of these curves isthat the curve can be translated and rotated by performing these operations on the control points.
Undesirable properties of Bézier curves are their numerical instability for large numbers of control points, and thefact that moving a single control point changes the global shape of the curve. The former is sometimes avoided bysmoothly patching together low-order Bézier curves. A generalization of the Bézier curve is the B-Spline.
See also B-Spline, NURBS Curve- Carlson-Levin Constant
- Carlson's Theorem
- Carlyle Circle
- Carmichael Condition
- Carmichael Function
- Carmichael Number
- Carmichael's Conjecture
- Carmichael Sequence
- Carmichael's Theorem
- Carmichael's Totient Function Conjecture
- Carnot's Polygon Theorem
- Carnot's Theorem
- Carotid-Kundalini Fractal
- Carotid-Kundalini Function
- Carry
- Carrying Capacity
- Cartan Matrix
- Cartan Relation
- Cartan Subgroup
- Cartan Torsion Coefficient
- Cartesian Coordinates
- Cartesian Ovals
- Cartesian Product
- Cartesian Trident
- Cartography