| 释义 |
Centroid (Triangle)The centroid (Center of Mass) of the Vertices of a Triangle is the point  (or (or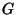 ) of intersection of the Triangle's three Medians, also called the Median Point(Johnson 1929, p. 249). The centroid is always in the interior of the Triangle, and has Trilinear Coordinates ) of intersection of the Triangle's three Medians, also called the Median Point(Johnson 1929, p. 249). The centroid is always in the interior of the Triangle, and has Trilinear Coordinates
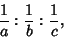 | (1) |
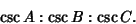 | (2) |
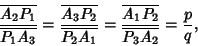 | (3) |
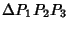 is is  (Johnson 1929, p. 250). (Johnson 1929, p. 250).
Pick an interior point  . The Triangles . The Triangles  , ,  , and , and  have equal areas Iff have equal areas Iff  corresponds to the centroid. The centroid is located one third of the way from each Vertex to the Midpoint of theopposite side. Each median divides the triangle into two equal areas; all the medians together divide it into six equalparts, and the lines from the Median Point to the Vertices divide the whole into three equivalent Triangles. In general, for any line in the plane of a Triangle corresponds to the centroid. The centroid is located one third of the way from each Vertex to the Midpoint of theopposite side. Each median divides the triangle into two equal areas; all the medians together divide it into six equalparts, and the lines from the Median Point to the Vertices divide the whole into three equivalent Triangles. In general, for any line in the plane of a Triangle  , ,
 | (4) |
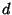 , , 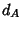 , , 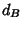 , and , and  are the distances from the centroid and Vertices to the line. A Triangle willbalance at the centroid, and along any line passing through the centroid. The Trilinear Polar of the centroid iscalled the Lemoine Axis. The Perpendiculars from the centroid areproportional to are the distances from the centroid and Vertices to the line. A Triangle willbalance at the centroid, and along any line passing through the centroid. The Trilinear Polar of the centroid iscalled the Lemoine Axis. The Perpendiculars from the centroid areproportional to  , ,
 | (5) |
 is the Area of the Triangle. Let is the Area of the Triangle. Let 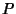 be an arbitrary point, the Vertices be be an arbitrary point, the Vertices be 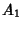 , , 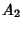 , and , and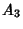 , and the centroid , and the centroid  . Then . Then
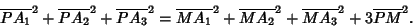 | (6) |
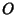 is the Circumcenter of the triangle's centroid, then is the Circumcenter of the triangle's centroid, then
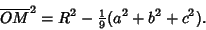 | (7) |
The centroid of the Perimeter of a Triangle is the triangle's Spieker Center (Johnson 1929, p. 249). See also Circumcenter, Euler Line, Exmedian Point, Incenter, Orthocenter
References
Carr, G. S. Formulas and Theorems in Pure Mathematics, 2nd ed. New York: Chelsea, p. 622, 1970.Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., p. 7, 1967. Dixon, R. Mathographics. New York: Dover, pp. 55-57, 1991. Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 173-176 and 249, 1929. Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994. Kimberling, C. ``Centroid.''http://cedar.evansville.edu/~ck6/tcenters/class/centroid.html.
|
![]() (or
(or![]() ) of intersection of the Triangle's three Medians, also called the Median Point(Johnson 1929, p. 249). The centroid is always in the interior of the Triangle, and has Trilinear Coordinates
) of intersection of the Triangle's three Medians, also called the Median Point(Johnson 1929, p. 249). The centroid is always in the interior of the Triangle, and has Trilinear Coordinates![]() . The Triangles
. The Triangles ![]() ,
, ![]() , and
, and ![]() have equal areas Iff
have equal areas Iff ![]() corresponds to the centroid. The centroid is located one third of the way from each Vertex to the Midpoint of theopposite side. Each median divides the triangle into two equal areas; all the medians together divide it into six equalparts, and the lines from the Median Point to the Vertices divide the whole into three equivalent Triangles. In general, for any line in the plane of a Triangle
corresponds to the centroid. The centroid is located one third of the way from each Vertex to the Midpoint of theopposite side. Each median divides the triangle into two equal areas; all the medians together divide it into six equalparts, and the lines from the Median Point to the Vertices divide the whole into three equivalent Triangles. In general, for any line in the plane of a Triangle ![]() ,
,