| 释义 |
Square Triangular NumberA number which is simultaneously Square and Triangular. The first few are1, 36, 1225, 41616, 1413721, 48024900, ... (Sloane's A001110), corresponding to 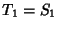 , , 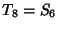 , ,  , , , , 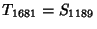 , ... (Pietenpol 1962), but there are an infinite number, as first shown byEuler , ... (Pietenpol 1962), but there are an infinite number, as first shown byEuler  in 1730 (Dickson 1952). in 1730 (Dickson 1952).
The general Formula for a square triangular number  is is 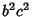 , where , where 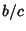 is the is the  th convergent to theContinued Fraction of th convergent to theContinued Fraction of  (Ball and Coxeter 1987, p. 59; Conway and Guy 1996). The first few are (Ball and Coxeter 1987, p. 59; Conway and Guy 1996). The first few are
 | (1) |
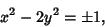 | (2) |
 denote the denote the  th Triangular Number and th Triangular Number and 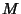 the the 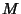 th Square Number, then th Square Number, then
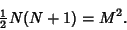 | (3) |
then gives the equation
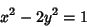 | (6) |
 | (7) |
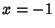 , 0, 1, 5, 6, and 85 (Guy 1994, p. 147). , 0, 1, 5, 6, and 85 (Guy 1994, p. 147).
A general Formula for square triangular numbers is
The square triangular numbers also satisfy the Recurrence Relation
with 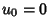 , , 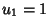 , where , where 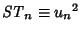 . A curious product formula for . A curious product formula for  is given by is given by
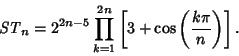 | (12) |
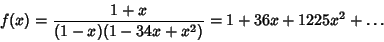 | (13) |
References
Allen, B. M. ``Squares as Triangular Numbers.'' Scripta Math. 20, 213-214, 1954.Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, 1987. Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 203-205, 1996. Dickson, L. E. A History of the Theory of Numbers, Vol. 2: Diophantine Analysis. New York: Chelsea, pp. 10, 16, and 27, 1952. Guy, R. K. ``Sums of Squares'' and ``Figurate Numbers.'' §C20 and §D3 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 136-138 and 147-150, 1994. Khatri, M. N. ``Triangular Numbers Which are Also Squares.'' Math. Student 27, 55-56, 1959. Pietenpol, J. L. ``Square Triangular Numbers.'' Problem E 1473. Amer. Math. Monthly 69, 168-169, 1962. Sierpinski, W. Teoria Liczb, 3rd ed. Warsaw, Poland: Monografie Matematyczne t. 19, p. 517, 1950. Sierpinski, W. ``Sur les nombres triangulaires carrés.'' Pub. Faculté d'Électrotechnique l'Université Belgrade, No. 65, 1-4, 1961. Sierpinski, W. ``Sur les nombres triangulaires carrés.'' Bull. Soc. Royale Sciences Liège, 30 ann., 189-194, 1961. Sloane, N. J. A. SequenceA001110/M5259in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Walker, G. W. ``Triangular Squares.'' Problem E 954. Amer. Math. Monthly 58, 568, 1951.
|
![]() ,
, ![]() ,
, ![]() ,
,![]() ,
, ![]() , ... (Pietenpol 1962), but there are an infinite number, as first shown byEuler
, ... (Pietenpol 1962), but there are an infinite number, as first shown byEuler ![]() in 1730 (Dickson 1952).
in 1730 (Dickson 1952).![]() is
is ![]() , where
, where ![]() is the
is the ![]() th convergent to theContinued Fraction of
th convergent to theContinued Fraction of ![]() (Ball and Coxeter 1987, p. 59; Conway and Guy 1996). The first few are
(Ball and Coxeter 1987, p. 59; Conway and Guy 1996). The first few are