“Tractrix”的概念、定义、习题解题方法及翻译-数学辞典

单词

Tractrix

释义

Tractrix

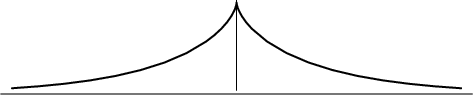
The tractrix is the Catenary Involute described by a point initially on the vertex. It is sometimes called the Tractory or Equitangential Curve. The tractrix was firststudied by Huygens in 1692, who gave it the name ``tractrix.'' Later, Leibniz, Johann Bernoulli, and others studied the curve.

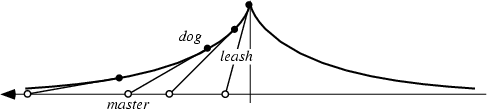
The tractrix arises from the following problem posed to Leibniz: What is the path of anobject starting off with a vertical offset when it is dragged along by a string of constant length being pulled along astraight horizontal line? By associating the object with a dog, the string with a leash, and the pull along a horizontalline with the dog's master, the curve has the descriptive name Hundkurve (hound curve) in German. Leibniz found thecurve using the fact that the axis is an asymptote to the tractrix (MacTutor Archive).

In Cartesian Coordinates the tractrix has equation

(1)

One parametric form is

			(2)
			(3)

The Arc Length, Curvature, and Tangential Angle are

			(4)
			(5)
			(6)

A second parametric form in terms of the Angle

of the straight line tangent to the tractrix is

			(7)
			(8)

(Gray 1993). This parameterization has Curvature

(9)

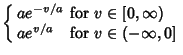A parameterization which traverses the tractrix with constant speed

is given by

			(10)

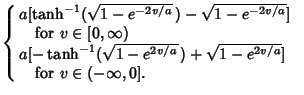			(11)

When a tractrix is rotated around its asymptote, a Pseudosphere results. This is a surface of constant NegativeCurvature. For a tractrix, the length of a Tangent from its point of contact to an asymptote is constant.The Area between the tractrix and its asymptote is finite.

See also Curvature, Dini's Surface, Mice Problem, Pseudosphere, Pursuit Curve,Tractroid

References

Geometry Center. ``The Tractrix.'' http://www.geom.umn.edu/zoo/diffgeom/pseudosphere/tractrix.html.

Gray, A. ``The Tractrix'' and ``The Evolute of a Tractrix is a Catenary.'' §3.5 and 5.3 in Modern Differential Geometry of Curves and Surfaces. Boca Raton, FL: CRC Press, pp. 46-50 and 80-81, 1993.

Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 199-200, 1972.

Lee, X. ``Tractrix.''http://www.best.com/~xah/SpecialPlaneCurves_dir/Tractrix_dir/tractrix.html.

Lockwood, E. H. ``The Tractrix and Catenary.'' Ch. 13 in A Book of Curves. Cambridge, England: Cambridge University Press, pp. 118-124, 1967.

MacTutor History of Mathematics Archive. ``Tractrix.''http://www-groups.dcs.st-and.ac.uk/~history/Curves/Tractrix.html.

Yates, R. C. ``Tractrix.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 221-224, 1952.

随便看

数学辞典收录了8975条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。