Unit Ring

A unit ring is a set together with two Binary Operators satisfying the following conditions:

1. Additive associativity: For all , ,

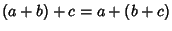2. Additive commutativity: For all , ,

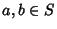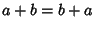3. Additive identity: There exists an element such that for all ,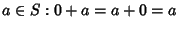

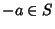4. Additive inverse: For every , there exists a such that ,

5. Multiplicative associativity: For all , ,

6. Multiplicative identity: There exists an element such that for all , ,

7. Left and right distributivity: For all , 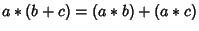and .

References

Rosenfeld, A. An Introduction to Algebraic Structures. New York: Holden-Day, 1968.

• Cone (Space)
• Cone-Sphere Intersection
• Confidence Interval
• Configuration
• Confluent Hypergeometric Differential Equation
• Confluent Hypergeometric Function
• Confluent Hypergeometric Function of the First Kind
• Confluent Hypergeometric Function of the Second Kind
• Confluent Hypergeometric Limit Function
• Confocal Conics
• Confocal Ellipsoidal Coordinates
• Confocal Parabolic Coordinates
• Confocal Paraboloidal Coordinates
• Conformal Latitude
• Conformal Map
• Conformal Solution
• Conformal Tensor
• Conformal Transformation
• Congruence
• Congruence Axioms
• Congruence (Geometric)
• Congruence Transformation
• Congruent
• Congruent Incircles Point
• Congruent Isoscelizers Point