| 释义 |
ErfThe ``error function'' encountered in integrating the Gaussian Distribution.
where Erfc is the complementary error function and  is the incomplete Gamma Function. It canalso be defined as a Maclaurin Series is the incomplete Gamma Function. It canalso be defined as a Maclaurin Series
 | (4) |
It is an Odd Function
 | (7) |
 | (8) |
 as as
 | (9) |
 | (10) |
 | (11) |
 is a Hermite Polynomial. The first Derivative is is a Hermite Polynomial. The first Derivative is
 | (12) |
 | (13) |
For 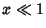 , erf may be computed from , erf may be computed from
(Acton 1990). For  , ,
Using Integration by Parts gives
so
 | (20) |
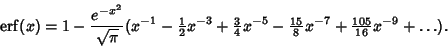 | (21) |
A Complex generalization of 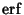 is defined as is defined as
See also Dawson's Integral,Erfc, Erfi, Gaussian Integral, Normal Distribution Function, Probability Integral
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Error Function'' and ``Repeated Integrals of the Error Function.'' §7.1-7.2 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 297-300, 1972.Acton, F. S. Numerical Methods That Work, 2nd printing. Washington, DC: Math. Assoc. Amer., p. 16, 1990. Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 568-569, 1985. Spanier, J. and Oldham, K. B. ``The Error Function  and Its Complement and Its Complement  .'' Ch. 40 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 385-393, 1987. .'' Ch. 40 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 385-393, 1987. |





![]() , erf may be computed from
, erf may be computed from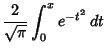





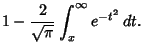

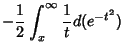
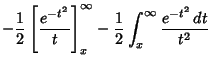


![]() is defined as
is defined as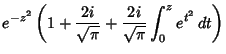
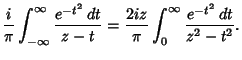
![]() and Its Complement
and Its Complement ![]() .'' Ch. 40 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 385-393, 1987.
.'' Ch. 40 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 385-393, 1987.