First Derivative Test
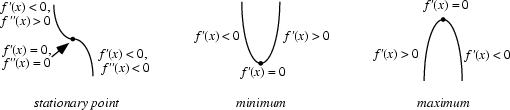
Suppose 
is Continuous at a Stationary Point 
.
- 1. If
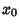on an Open Interval extending left from and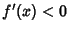 
on an Open Intervalextending right from 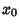, then has a Relative Maximum (possibly a Global Maximum) at 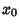. - 2. If 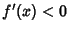on an Open Interval extending left from 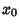and on an Open Interval extending rightfrom 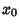, then has a Relative Minimum (possibly a Global Minimum) at .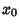
- 3. If
has the same sign on an Open Interval extending left from 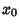and on an Open Intervalextending right from 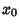, then does not have a Relative Extremum at .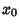
References
Abramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 14, 1972.
- Separation of Variables
- Separation Theorem
- Separatrix
- Septendecillion
- Septillion
- Sequence
- Sequency
- Sequency Function
- Sequential Graph
- Series
- Series Expansion
- Series Inversion
- Series Multisection
- Series Reversion
- Serpentine Curve
- Serret-Frenet Formulas
- Set
- Set Difference
- Set Partition
- Set Theory
- Sexagesimal
- Sexdecillion
- Sextic Equation
- Sextic Surface
- Sextillion