Frobenius Method
If ![]() is an ordinary point of the Ordinary Differential Equation, expand
is an ordinary point of the Ordinary Differential Equation, expand ![]() in a Taylor Series about
in a Taylor Series about ![]() , letting
, letting
 | (1) |
 | (2) | ||
 | (3) | ||
 | |||
| (4) |
If
| (5) |
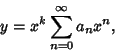 | (6) |
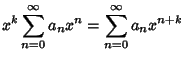 | (7) | ||
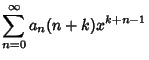 | (8) | ||
 | (9) |
Now, plug
Fuchs's Theorem guarantees that at least one Power series solution will be obtained when applying the Frobeniusmethod if the expansion point is an ordinary, or regular, Singular Point. For a regularSingular Point, a Laurent Series expansion can also be used. Expand ![]() in aLaurent Series, letting
in aLaurent Series, letting
| (10) |
References
Arfken, G. ``Series Solutions--Frobenius' Method.'' §8.5 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 454-467, 1985.
- Trigonal Dipyramid
- Trigonal Dodecahedron
- Trigonometric Functions
- Trigonometric Series
- Trigonometric Substitution
- Trigonometry
- Trigonometry Values--0
- Trigonometry Values Pi
- Trigonometry Values Pi/10
- Trigonometry Values Pi/11
- Trigonometry Values Pi/12
- Trigonometry Values Pi/15
- Trigonometry Values Pi/16
- Trigonometry Values Pi/17
- Trigonometry Values Pi/18
- Trigonometry Values Pi/2
- Trigonometry Values Pi/20
- Trigonometry Values Pi/3
- Cycle (Circle)
- Cycle Graph
- Cycle (Map)
- Cycle (Permutation)
- Cyclic Graph
- Cyclic Group
- Cyclic Hexagon