| 释义 |
Greatest Common DivisorThe greatest common divisor of  and and 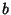 GCD( GCD( ), sometimes written ), sometimes written 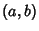 , is the largest Divisor common to , is the largest Divisor common to  and and 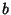 . Symbolically, let . Symbolically, let
Then the greatest common divisor is given by
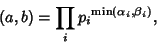 | (3) |
 | (4) |
 | (5) |
 | (6) |
 | (7) |
If  and and 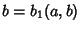 , then , then
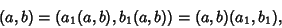 | (8) |
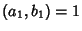 and and  and and  are said to be Relatively Prime. The GCD is also Idempotent are said to be Relatively Prime. The GCD is also Idempotent
 | (9) |
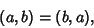 | (10) |
 | (11) |
The probability that two Integers picked at random are Relatively Prime is 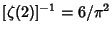 ,where ,where 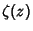 is the Riemann Zeta Function. Polezzi (1997) observed that is the Riemann Zeta Function. Polezzi (1997) observed that 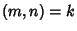 , where , where  is the number ofLattice Points in the Plane on the straight Line connecting theVectors (0, 0) and is the number ofLattice Points in the Plane on the straight Line connecting theVectors (0, 0) and 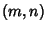 (excluding (excluding 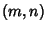 itself). This observation is intimately connected with theprobability of obtaining Relatively Prime integers, and also with the geometric interpretation of a ReducedFraction itself). This observation is intimately connected with theprobability of obtaining Relatively Prime integers, and also with the geometric interpretation of a ReducedFraction  as a string through a Lattice of points with ends at (1,0) and as a string through a Lattice of points with ends at (1,0) and 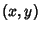 . The pegs it presses against . The pegs it presses against give alternate Convergents give alternate Convergents  of the Continued Fraction for of the Continued Fraction for  , while theother Convergents are obtained from the pegs it presses against with the initial end at (0, 1). , while theother Convergents are obtained from the pegs it presses against with the initial end at (0, 1).
Knuth showed that
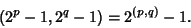 | (12) |
The extended greatest common divisor of two Integers  and and  can be defined as the greatest commondivisor can be defined as the greatest commondivisor 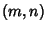 of of  and and  which also satisfies the constraint which also satisfies the constraint  for for  and and 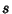 given Integers. It isused in solving Linear Diophantine Equations. given Integers. It isused in solving Linear Diophantine Equations. See also Bezout Numbers, Euclidean Algorithm, Least Prime Factor
References
Polezzi, M. ``A Geometrical Method for Finding an Explicit Formula for the Greatest Common Divisor.'' Amer. Math. Monthly 104, 445-446, 1997. |
![]() and
and ![]() GCD(
GCD(![]() ), sometimes written
), sometimes written ![]() , is the largest Divisor common to
, is the largest Divisor common to ![]() and
and ![]() . Symbolically, let
. Symbolically, let![]() and
and ![]() , then
, then![]() ,where
,where ![]() is the Riemann Zeta Function. Polezzi (1997) observed that
is the Riemann Zeta Function. Polezzi (1997) observed that ![]() , where
, where ![]() is the number ofLattice Points in the Plane on the straight Line connecting theVectors (0, 0) and
is the number ofLattice Points in the Plane on the straight Line connecting theVectors (0, 0) and ![]() (excluding
(excluding ![]() itself). This observation is intimately connected with theprobability of obtaining Relatively Prime integers, and also with the geometric interpretation of a ReducedFraction
itself). This observation is intimately connected with theprobability of obtaining Relatively Prime integers, and also with the geometric interpretation of a ReducedFraction ![]() as a string through a Lattice of points with ends at (1,0) and
as a string through a Lattice of points with ends at (1,0) and ![]() . The pegs it presses against
. The pegs it presses against![]() give alternate Convergents
give alternate Convergents ![]() of the Continued Fraction for
of the Continued Fraction for ![]() , while theother Convergents are obtained from the pegs it presses against with the initial end at (0, 1).
, while theother Convergents are obtained from the pegs it presses against with the initial end at (0, 1).![]() and
and ![]() can be defined as the greatest commondivisor
can be defined as the greatest commondivisor ![]() of
of ![]() and
and ![]() which also satisfies the constraint
which also satisfies the constraint ![]() for
for ![]() and
and ![]() given Integers. It isused in solving Linear Diophantine Equations.
given Integers. It isused in solving Linear Diophantine Equations.