Hilbert Space
A Hilbert space is Vector Space 
with an Inner Product 
such that the Norm defined by
turns
into a Complete Metric Space. If the Inner Product does not so define aNorm, it is instead known as an Inner Product Space.Examples of Finite-dimensional Hilbert spaces include
- 1. The Real Numbers
with 
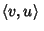the vector Dot Product of 
and 
. - 2. The Complex Numbers
with 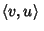the vector Dot Productof and the Complex Conjugate of .
, the Set of all Functions
such that the Integral of 
over the whole Real Line is Finite. In this case,the Inner Product is
A Hilbert space is always a Banach Space, but the converse need not hold.
See also Banach Space, L2-Norm, L2-Space, Liouville Space, Parallelogram Law, Vector Space
References
Sansone, G. ``Elementary Notions of Hilbert Space.'' §1.3 in Orthogonal Functions, rev. English ed. New York: Dover, pp. 5-10, 1991. Stone, M. H. Linear Transformations in Hilbert Space and Their Applications Analysis. Providence, RI: Amer. Math. Soc., 1932.
- Skeleton
- Skeleton Division
- Skew Conic
- Skewes Number
- Skew Field
- Skew Lines
- Skewness
- Skew Polyomino
- Skew Quadrilateral
- Skew Symmetric Matrix
- Sklar's Theorem
- Skolem-Mahler-Lerch Theorem
- Skolem Paradox
- Skolem Sequence
- Slant Height
- Slice Knot
- Slide Move
- Slide Rule
- Slightly Defective Number
- Slightly Excessive Number
- Slip Knot
- Slope
- Slothouber-Graatsma Puzzle
- Slutzky-Yule Effect
- Sluze Pearls