| 释义 |
Khintchine's ConstantN.B. A detailed on-line essay by S. Finchwas the starting point for this entry.
Let
 | (1) |
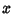 , where the numbers , where the numbers 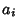 are PartialQuotients. Khintchine (1934) considered the limit of the Geometric Mean are PartialQuotients. Khintchine (1934) considered the limit of the Geometric Mean
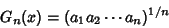 | (2) |
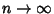 . Amazingly enough, this limit is a constant independent of . Amazingly enough, this limit is a constant independent of 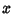 --except if --except if 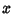 belongs to a setof Measure 0-given by belongs to a setof Measure 0-given by
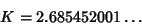 | (3) |
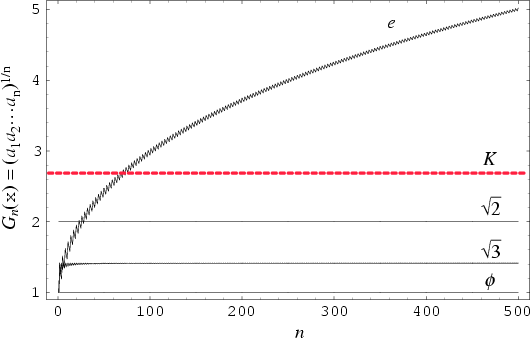
 are plotted above for are plotted above for 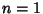 to 500 and to 500 and 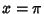 , ,  , , 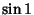 , the Euler-Mascheroni Constant , the Euler-Mascheroni Constant 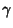 , and the Copeland-Erdös Constant.Real Numbers , and the Copeland-Erdös Constant.Real Numbers 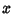 for which for which 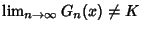 include include 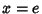 , , 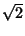 , ,  , andthe Golden Ratio , andthe Golden Ratio 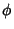 , plotted below. , plotted below.
The Continued Fraction for  is [2, 1, 2, 5, 1, 1, 2, 1, 1, ...] (Sloane's A002211). It is not known if is [2, 1, 2, 5, 1, 1, 2, 1, 1, ...] (Sloane's A002211). It is not known if  isIrrational, let alone Transcendental. Bailey et al. (1995) havecomputed isIrrational, let alone Transcendental. Bailey et al. (1995) havecomputed  to 7350 Digits. to 7350 Digits.
Explicit expressions for  include include
where 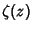 is the Riemann Zeta Function and is the Riemann Zeta Function and
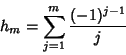 | (7) |
 | (8) |
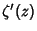 is the Derivative of the Riemann Zeta Function. An extremely rapidly converging sum also dueto Gosper is is the Derivative of the Riemann Zeta Function. An extremely rapidly converging sum also dueto Gosper is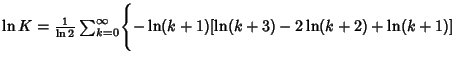 | | 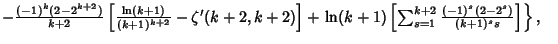 | (9) |
where 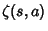 is the Hurwitz Zeta Function. is the Hurwitz Zeta Function.
Khintchine's constant is also given by the integral
 | (10) |
If 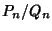 is the is the  th Convergent of the Continued Fraction of th Convergent of the Continued Fraction of 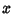 , then , then
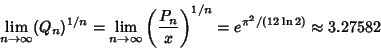 | (11) |
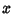 (Lévy 1936, Finch). This number is sometimes called the Lévy Constant, and the argument of the exponential is sometimes called the Khintchine-LévyConstant. (Lévy 1936, Finch). This number is sometimes called the Lévy Constant, and the argument of the exponential is sometimes called the Khintchine-LévyConstant.
Define the following quantity in terms of the  th partial quotient th partial quotient 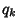 , ,
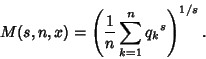 | (12) |
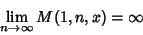 | (13) |
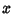 (Khintchine, Knuth 1981, Finch), and (Khintchine, Knuth 1981, Finch), and
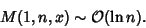 | (14) |
 , the limiting value , the limiting value
 | (15) |
 with probability 1 (Rockett and Szüsz 1992, Khintchine 1997).See also Continued Fraction, Convergent, Khintchine-Lévy Constant,Lévy Constant, Partial Quotient, Simple Continued Fraction with probability 1 (Rockett and Szüsz 1992, Khintchine 1997).See also Continued Fraction, Convergent, Khintchine-Lévy Constant,Lévy Constant, Partial Quotient, Simple Continued Fraction
References
Bailey, D. H.; Borwein, J. M.; and Crandall, R. E. ``On the Khintchine Constant.'' Math. Comput. 66, 417-431, 1997.Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/khntchn/khntchn.html Kac, M. Statistical Independence and Probability, Analysts and Number Theory. Providence, RI: Math. Assoc. Amer., 1959. Khinchin, A. Ya. Continued Fractions. New York: Dover, 1997. Knuth, D. E. Exercise 24 in The Art of Computer Programming, Vol. 2: Seminumerical Algorithms, 2nd ed. Reading, MA: Addison-Wesley, p. 604, 1981. Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 46, 1983. Lehmer, D. H. ``Note on an Absolute Constant of Khintchine.'' Amer. Math. Monthly 46, 148-152, 1939. Phillipp, W. ``Some Metrical Theorems in Number Theory.'' Pacific J. Math. 20, 109-127, 1967. Plouffe, S. ``Plouffe's Inverter: Table of Current Records for the Computationof Constants.'' http://www.lacim.uqam.ca/pi/records.html. Rockett, A. M. and Szüsz, P. Continued Fractions. Singapore: World Scientific, 1992. Shanks, D. and Wrench, J. W. ``Khintchine's Constant.'' Amer. Math. Monthly 66, 148-152, 1959. Sloane, N. J. A. SequencesA002210/M1564and A002211/M0118in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Vardi, I. ``Khinchin's Constant.'' §8.4 in Computational Recreations in Mathematica. Reading, MA: Addison-Wesley, pp. 163-171, 1991. Wrench, J. W. ``Further Evaluation of Khintchine's Constant.'' Math. Comput. 14, 370-371, 1960.
|


![]() is [2, 1, 2, 5, 1, 1, 2, 1, 1, ...] (Sloane's A002211). It is not known if
is [2, 1, 2, 5, 1, 1, 2, 1, 1, ...] (Sloane's A002211). It is not known if ![]() isIrrational, let alone Transcendental. Bailey et al. (1995) havecomputed
isIrrational, let alone Transcendental. Bailey et al. (1995) havecomputed ![]() to 7350 Digits.
to 7350 Digits.![]() include
include

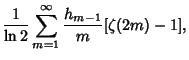
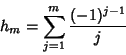

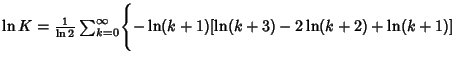
![]() is the
is the ![]() th Convergent of the Continued Fraction of
th Convergent of the Continued Fraction of ![]() , then
, then![]() th partial quotient
th partial quotient ![]() ,
,