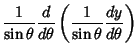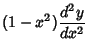| 释义 |
Legendre Differential EquationThe second-order Ordinary Differential Equation
 | (1) |
 | (2) |
 . The Legendre differentialequation has Regular Singular Points at . The Legendre differentialequation has Regular Singular Points at  , 1, and , 1, and  . It can be solved using aseries expansion, . It can be solved using aseries expansion,
Plugging in,
 | (6) |
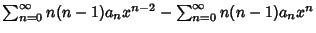 | |  | (7) |
 | |  | (8) |
 | |  | (9) |
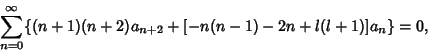 | (10) |
 | (11) |
Therefore,
so the Even solution is
 | (16) |
Similarly, the Odd solution is
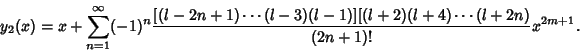 | (17) |
If  is an Even Integer, the series is an Even Integer, the series  reduces to a Polynomial of degree reduces to a Polynomial of degree  with only EvenPowers of with only EvenPowers of  and the series and the series  diverges. If diverges. If  is an Odd Integer, the series is an Odd Integer, the series  reducesto a Polynomial of degree reducesto a Polynomial of degree  with only Odd Powers of with only Odd Powers of  and the series and the series  diverges. Thegeneral solution for an Integer diverges. Thegeneral solution for an Integer  is given by the Legendre Polynomials is given by the Legendre Polynomials
 | (18) |
 is chosen so that is chosen so that  . If the variable . If the variable  is replaced by is replaced by  , then the Legendredifferential equation becomes , then the Legendredifferential equation becomes
 | (19) |
 . .
The associated Legendre differential equation is
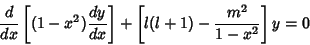 | (20) |
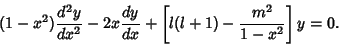 | (21) |
 , first establishthe identities , first establishthe identities
 | (22) |
 | (23) |
and
 | (25) |
Plugging (22) into (26) and the result back into (21) gives
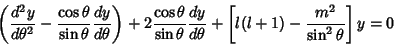 | (27) |
 | (28) |
References
Abramowitz, M. and Stegun, C. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 332, 1972.
|




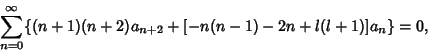








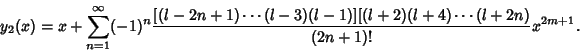
![]() is an Even Integer, the series
is an Even Integer, the series ![]() reduces to a Polynomial of degree
reduces to a Polynomial of degree ![]() with only EvenPowers of
with only EvenPowers of ![]() and the series
and the series ![]() diverges. If
diverges. If ![]() is an Odd Integer, the series
is an Odd Integer, the series ![]() reducesto a Polynomial of degree
reducesto a Polynomial of degree ![]() with only Odd Powers of
with only Odd Powers of ![]() and the series
and the series ![]() diverges. Thegeneral solution for an Integer
diverges. Thegeneral solution for an Integer ![]() is given by the Legendre Polynomials
is given by the Legendre Polynomials