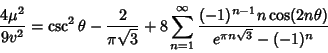| 释义 |
Lemniscate FunctionThe lemniscate functions arise in rectifying the Arc Length of the Lemniscate. The lemniscate functionswere first studied by Jakob Bernoulli  and G. Fagnano. A historical account is given byAyoub (1984), and an extensive discussion by Siegel (1969). The lemniscate functions were the first functions defined byinversion of an integral, which was first done by Gauß. and G. Fagnano. A historical account is given byAyoub (1984), and an extensive discussion by Siegel (1969). The lemniscate functions were the first functions defined byinversion of an integral, which was first done by Gauß. 
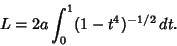 | (1) |
where
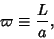 | (4) |
There is an identity connecting 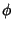 and and 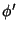 since since
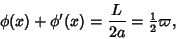 | (7) |
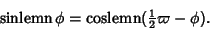 | (8) |
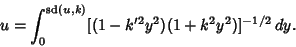 | (9) |
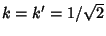 , then , then
Let 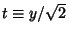 so so 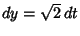 , ,
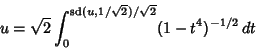 | (11) |
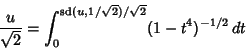 | (12) |
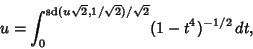 | (13) |
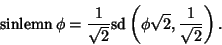 | (14) |
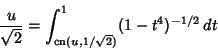 | (16) |
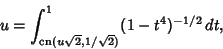 | (17) |
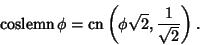 | (18) |
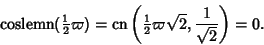 | (19) |
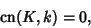 | (20) |
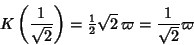 | (21) |
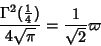 | (22) |
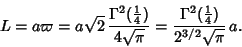 | (23) |
By expanding 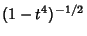 in a Binomial Series and integrating term by term, the arcsinlemn function can bewritten in a Binomial Series and integrating term by term, the arcsinlemn function can bewritten
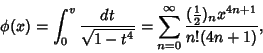 | (24) |
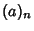 is the Ramanujan is the Ramanujan  gave the following inversion Formula for gave the following inversion Formula for 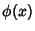 . If . If
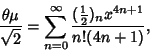 | (25) |
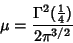 | (26) |
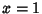 and and 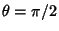 , and , and
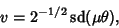 | (27) |
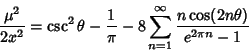 | (28) |
 also showed that if also showed that if 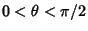 , then , then
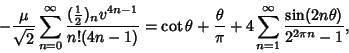 | (29) |
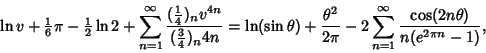 | (30) |
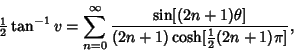 | (31) |
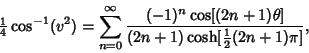 | (32) |
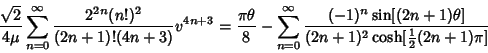 | (33) |
A generalized version of the lemniscate function can be defined byletting 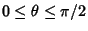 and and 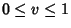 . Write . Write
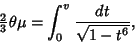 | (34) |
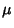 is the constant obtained by setting is the constant obtained by setting 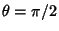 and and 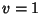 . Then . Then
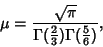 | (35) |
 showed showed
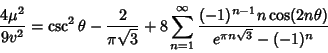 | (36) |
References
Ayoub, R. ``The Lemniscate and Fagnano's Contributions to Elliptic Integrals.'' Arch. Hist. Exact Sci. 29, 131-149, 1984.Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 245, and 247-255, 258-260, 1994. Siegel, C. L. Topics in Complex Function Theory, Vol. 1. New York: Wiley, 1969.
|
![]() and G. Fagnano. A historical account is given byAyoub (1984), and an extensive discussion by Siegel (1969). The lemniscate functions were the first functions defined byinversion of an integral, which was first done by Gauß.
and G. Fagnano. A historical account is given byAyoub (1984), and an extensive discussion by Siegel (1969). The lemniscate functions were the first functions defined byinversion of an integral, which was first done by Gauß. ![]()
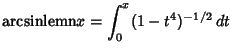

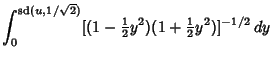


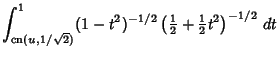
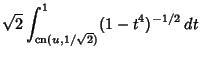
![]() in a Binomial Series and integrating term by term, the arcsinlemn function can bewritten
in a Binomial Series and integrating term by term, the arcsinlemn function can bewritten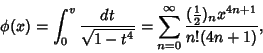
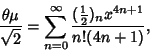
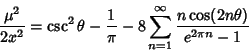
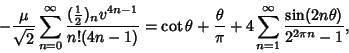
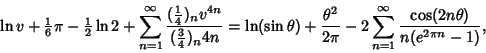
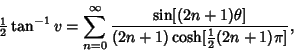
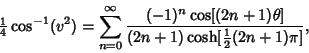
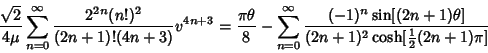
![]() and
and ![]() . Write
. Write