| 释义 |
Lucas NumberThe numbers produced by the  recurrence in the Lucas Sequence with recurrence in the Lucas Sequence with 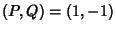 are called Lucas numbers. Theyare the companions to the Fibonacci Numbers are called Lucas numbers. Theyare the companions to the Fibonacci Numbers  and satisfy the same recurrence and satisfy the same recurrence
 | (1) |
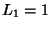 , , 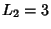 . The first few are 1, 3, 4, 7, 11, 18, 29, 47, 76, 123, ... (Sloane's A000204). . The first few are 1, 3, 4, 7, 11, 18, 29, 47, 76, 123, ... (Sloane's A000204).
In terms of the Fibonacci Numbers,
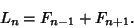 | (2) |
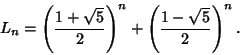 | (3) |
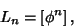 | (4) |
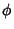 is the Golden Ratio and is the Golden Ratio and  denotes the Nint function. Given denotes the Nint function. Given  , ,
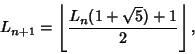 | (5) |
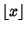 is the Floor Function, is the Floor Function,
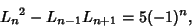 | (6) |
 | (7) |
 be a Prime be a Prime  and and 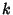 be a Positive Integer. Then be a Positive Integer. Then 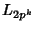 ends in a 3 (Honsberger 1985, p. 113).Analogs of the Cesàro identities for Fibonacci Numbers are ends in a 3 (Honsberger 1985, p. 113).Analogs of the Cesàro identities for Fibonacci Numbers are
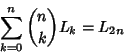 | (8) |
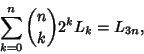 | (9) |
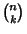 is a Binomial Coefficient. is a Binomial Coefficient.
 ( ( Divides Divides 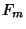 ) Iff ) Iff 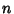 Divides into Divides into 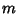 an Even number of times. an Even number of times. 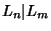 Iff Iff 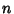 divides into divides into 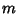 an Odd number of times. an Odd number of times.  always ends in 2 (Honsberger 1985, p. 137). always ends in 2 (Honsberger 1985, p. 137).
Defining
 | (10) |
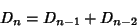 | (11) |
The number of ways of picking a set (including the Empty Set) from the numbers 1, 2, ..., 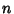 without pickingtwo consecutive numbers (where 1 and without pickingtwo consecutive numbers (where 1 and 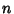 are now consecutive) is are now consecutive) is  (Honsberger 1985, p. 122). (Honsberger 1985, p. 122).
The only Square Numbers in the Lucas sequence are 1 and 4, as proved by John H. E. Cohn (Alfred 1964). The only Triangular Lucas numbers are 1, 3, and 5778 (Ming 1991). The only Lucas CubicNumber is 1. The first few Lucas Primes  occur for occur for 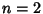 , 4, 5, 7, 8, 11, 13, 16, 17, 19, 31, 37, 41, 47, 53, 61, 71,79, 113, 313, 353, ... (Dubner and Keller 1999, Sloane's A001606). , 4, 5, 7, 8, 11, 13, 16, 17, 19, 31, 37, 41, 47, 53, 61, 71,79, 113, 313, 353, ... (Dubner and Keller 1999, Sloane's A001606). See also Fibonacci Number
References
Alfred, Brother U. ``On Square Lucas Numbers.'' Fib. Quart. 2, 11-12, 1964.Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, pp. 94-101, 1987. Brillhart, J.; Montgomery, P. L.; and Solverman, R. D. ``Tables of Fibonacci and Lucas Factorizations.'' Math. Comput. 50, 251-260 and S1-S15, 1988. Brown, J. L. Jr. ``Unique Representation of Integers as Sums of Distinct Lucas Numbers.'' Fib. Quart. 7, 243-252, 1969. Dubner, H. and Keller, W. ``New Fibonacci and Lucas Primes.'' Math. Comput. 68, 417-427 and S1-S12, 1999. Guy, R. K. ``Fibonacci Numbers of Various Shapes.'' §D26 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 194-195, 1994. Hoggatt, V. E. Jr. The Fibonacci and Lucas Numbers. Boston, MA: Houghton Mifflin, 1969. Honsberger, R. ``A Second Look at the Fibonacci and Lucas Numbers.'' Ch. 8 in Mathematical Gems III. Washington, DC: Math. Assoc. Amer., 1985. Leyland, P. ftp://sable.ox.ac.uk/pub/math/factors/lucas.Z. Ming, L. ``On Triangular Lucas Numbers.'' Applications of Fibonacci Numbers, Vol. 4 (Ed. G. E. Bergum, A. N. Philippou, and A. F. Horadam). Dordrecht, Netherlands: Kluwer, pp. 231-240, 1991. Sloane, N. J. A. SequencesA000204/M2341and A001606/M0961in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. |
![]() recurrence in the Lucas Sequence with
recurrence in the Lucas Sequence with ![]() are called Lucas numbers. Theyare the companions to the Fibonacci Numbers
are called Lucas numbers. Theyare the companions to the Fibonacci Numbers ![]() and satisfy the same recurrence
and satisfy the same recurrence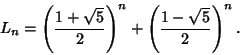
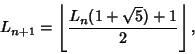

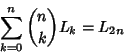
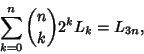
![]() (
(![]() Divides
Divides ![]() ) Iff
) Iff ![]() Divides into
Divides into ![]() an Even number of times.
an Even number of times. ![]() Iff
Iff ![]() divides into
divides into ![]() an Odd number of times.
an Odd number of times. ![]() always ends in 2 (Honsberger 1985, p. 137).
always ends in 2 (Honsberger 1985, p. 137).
![]() without pickingtwo consecutive numbers (where 1 and
without pickingtwo consecutive numbers (where 1 and ![]() are now consecutive) is
are now consecutive) is ![]() (Honsberger 1985, p. 122).
(Honsberger 1985, p. 122).![]() occur for
occur for ![]() , 4, 5, 7, 8, 11, 13, 16, 17, 19, 31, 37, 41, 47, 53, 61, 71,79, 113, 313, 353, ... (Dubner and Keller 1999, Sloane's A001606).
, 4, 5, 7, 8, 11, 13, 16, 17, 19, 31, 37, 41, 47, 53, 61, 71,79, 113, 313, 353, ... (Dubner and Keller 1999, Sloane's A001606).