“Magic Square”的概念、定义、习题解题方法及翻译-数学辞典

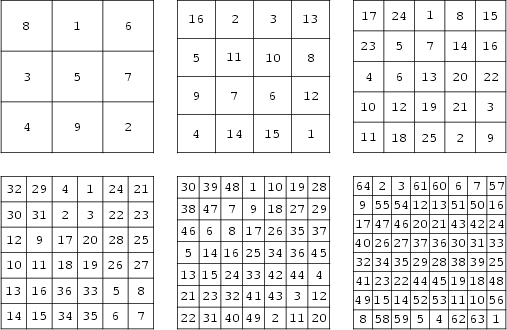
A (normal) magic square consists of the distinct Positive Integers 1, 2, ...,

such that thesum of the

numbers in any horizontal, vertical, or main diagonal line is always the same Magic Constant

The Magic Constant for an

th order magic square starting with an Integer

and with entries in an increasingArithmetic Series with difference

between terms is

A square that fails to be magic only because one or both of the main diagonal sums do not equal the Magic Constant iscalled a Semimagic Square. If all diagonals (including those obtained by wrapping around) of a magic square sumto the Magic Constant, the square is said to be a Panmagic Square (also called a Diabolical Square orPandiagonal Square). If replacing each number

by its square

produces another magic square, thesquare is said to be a Bimagic Square (or Doubly Magic Square). If a square is magic for

,and

, it is called a Trebly Magic Square. If all pairs of numbers symmetrically opposite the center sum to

, the square is said to be an Associative Magic Square.

Kraitchik (1942) gives general techniques of constructing Even and Odd squares of order

. For

Odd, a verystraightforward technique known as the Siamese method can be used, as illustrated above (Kraitchik 1942, pp. 148-149). Itbegins by placing a 1 in any location (in the center square of the top row in the above example), then incrementally placingsubsequent numbers in the square one unit above and to the right. The counting is wrapped around, so that falling off thetop returns on the bottom and falling off the right returns on the left. When a square is encountered which is alreadyfilled, the next number is instead placed below the previous one and the method continues as before. The method, alsocalled de la Loubere's method, is purported to have been first reported in the West when de la Loubere returned to Franceafter serving as ambassador to Siam.

A generalization of this method uses an ``ordinary vector''

which gives the offset for each noncolliding move anda ``break vector''

which gives the offset to introduce upon a collision. The standard Siamese method therefore hasordinary vector (1,

and break vector (0, 1). In order for this to produce a magic square, each break move must end upon an unfilled cell. Special classes of magic squares can be constructed by considering the absolute sums

, and

. Call the set of these numbers the sumdiffs (sums and differences). If all sumdiffs are Relatively Prime to

and the square is a magic square, then the square is also aPanmagic Square. This theory originated with de la Hire. The following table gives the sumdiffs for particularchoices of ordinary and break vectors.

Ordinary Vector	Break Vector	Sumdiffs	Magic Squares	Panmagic Squares
(1, )	(0, 1)	(1, 3)		none
(1, )	(0, 2)	(0, 2)		none
(2, 1)	(1, )	(1, 2, 3, 4)		none
(2, 1)	(1, )	(0, 1, 2, 3)
(2, 1)	(1, 0)	(0, 1, 2)		none
(2, 1)	(1, 2)	(0, 1, 2, 3)		none

A second method for generating magic squares of Odd order has been discussed by J. H. Conway under the name of the``lozenge'' method. As illustrated above, in this method, the Odd numbers are built up along diagonal lines in theshape of a Diamond in the central part of the square. The Even numbers which were missed are then addedsequentially along the continuation of the diagonal obtained by wrapping around the square until the wrapped diagonalreaches its initial point. In the above square, the first diagonal therefore fills in 1, 3, 5, 2, 4, the second diagonalfills in 7, 9, 6, 8, 10, and so on.

An elegant method for constructing magic squares of Doubly Even order

is to draw

sthrough each

subsquare and fill all squares in sequence. Then replace each entry

on a crossed-offdiagonal by

or, equivalently, reverse the order of the crossed-out entries. Thus in the above example for

, the crossed-out numbers are originally 1, 4, ..., 61, 64, so entry 1 is replaced with 64, 4 with 61, etc.

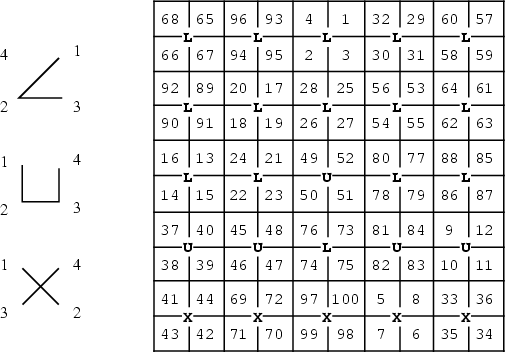
A very elegant method for constructing magic squares of Singly Even order

with

(there is no magic square of order 2) is due to J. H. Conway, who calls it the ``LUX'' method. Create an array consistingof

rows of

s, 1 row of Us, and

rows of

s, all of length

. Interchange the middle U with the Labove it. Now generate the magic square of order

using the Siamese method centered on the array of letters (startingin the center square of the top row), but fill each set of four squares surrounding a letter sequentially according to theorder prescribed by the letter. That order is illustrated on the left side of the above figure, and the completedsquare is illustrated to the right. The ``shapes'' of the letters L, U, and X naturally suggest the filling order, hencethe name of the algorithm.

It is an unsolved problem to determine the number of magic squares of an arbitrary order, but the number of distinct magicsquares (excluding those obtained by rotation and reflection) of order

, 2, ... are 1, 0, 1, 880, 275305224, ...(Sloane's A006052; Madachy 1979, p. 87). The 880 squares of order four were enumerated by Frenicle de Bessy in the seventeenthcentury, and are illustrated in Berlekamp et al. (1982, pp. 778-783). The number of

squares is not known.

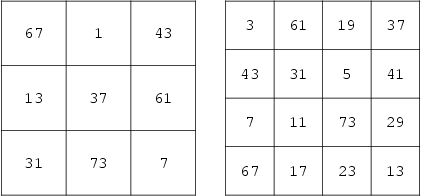
The above magic squares consist only of Primes and were discovered by E. Dudeney (1970) and A. W. Johnson, Jr. (Dewdney 1988). Madachy (1979, pp. 93-96) and Rivera discuss other magic squares composed of Primes.

Benjamin Franklin constructed the above

Panmagic Square having Magic Constant 260. Any half-row orhalf-column in this square totals 130, and the four corners plus the middle total 260. In addition, bent diagonals (such as52-3-5-54-10-57-63-16) also total 260 (Madachy 1979, p. 87).

In addition to other special types of magic squares, a

square whose entries are consecutive Primes, illustrated above, has been discovered by H. Nelson (Rivera). Variations on magic squares can also be constructed usingletters (either in defining the square or as entries in it), such as the Alphamagic Square and TemplarMagic Square.

Various numerological properties have also been associated with magic squares. Pivari associates the squares illustratedabove with Saturn, Jupiter, Mars, the Sun, Venus, Mercury, and the Moon, respectively. Attractive patterns are obtainedby connecting consecutive numbers in each of the squares (with the exception of the Sun magic square).

Abe, G. ``Unsolved Problems on Magic Squares.'' Disc. Math. 127, 3-13, 1994.

Alejandre, S. ``Suzanne Alejandre's Magic Squares.'' http://forum.swarthmore.edu/alejandre/magic.square.html.

Ball, W. W. R. and Coxeter, H. S. M. ``Magic Squares.'' Ch. 7 in Mathematical Recreations and Essays, 13th ed. New York: Dover, 1987.

Barnard, F. A. P. ``Theory of Magic Squares and Cubes.'' Memoirs Natl. Acad. Sci. 4, 209-270, 1888.

Benson, W. H. and Jacoby, O. New Recreations with Magic Squares. New York: Dover, 1976.

Berlekamp, E. R.; Conway, J. H; and Guy, R. K. Winning Ways, For Your Mathematical Plays, Vol. 2: Games in Particular. London: Academic Press, 1982.

Dewdney, A. K. ``Computer Recreations: How to Pan for Primes in Numerical Gravel.'' Sci. Amer. 259, pp. 120-123, July 1988.

Gardner, M. ``Magic Squares.'' Ch. 12 in The Second Scientific American Book of Mathematical Puzzles & Diversions: A New Selection. New York: Simon and Schuster, 1961.

Gardner, M. ``Magic Squares and Cubes.'' Ch. 17 in Time Travel and Other Mathematical Bewilderments. New York: W. H. Freeman, 1988.

Heinz, H. ``Magic Squares.'' http://www.geocities.com/CapeCanaveral/Launchpad/4057/magicsquare.htm.

Hirayama, A. and Abe, G. Researches in Magic Squares. Osaka, Japan: Osaka Kyoikutosho, 1983.

Horner, J. ``On the Algebra of Magic Squares, I., II., and III.'' Quart. J. Pure Appl. Math. 11, 57-65, 123-131, and 213-224, 1871.

Hunter, J. A. H. and Madachy, J. S. ``Mystic Arrays.'' Ch. 3 in Mathematical Diversions. New York: Dover, pp. 23-34, 1975.

Kanada, Y. ``Magic Square Page.'' http://www.st.rim.or.jp/~kanada/puzzles/magic-square.html.

Kraitchik, M. ``Magic Squares.'' Ch. 7 in Mathematical Recreations. New York: Norton, pp. 142-192, 1942.

Lei, A. ``Magic Square, Cube, Hypercube.'' http://www.cs.ust.hk/~philipl/magic/.

Madachy, J. S. ``Magic and Antimagic Squares.'' Ch. 4 in Madachy's Mathematical Recreations. New York: Dover, pp. 85-113, 1979.

Pappas, T. ``Magic Squares,'' ``The `Special' Magic Square,'' ``The Pyramid Method for Making Magic Squares,'' ``Ancient Tibetan Magic Square,'' ``Magic `Line.','' and ``A Chinese Magic Square.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 82-87, 112, 133, 169, and 179, 1989.

Pivari, F. ``Nice Examples.'' http://www.geocities.com/CapeCanaveral/Lab/3469/examples.html.

Pivari, F. ``Simple Magic Square Checker and GIF Maker.'' http://www.geocities.com/CapeCanaveral/Lab/3469/squaremaker.html.

Rivera, C. ``Problems & Puzzles (Puzzles): Magic Squares with Consecutive Primes.'' http://www.sci.net.mx/~crivera/puzzles/puzz_003.htm.

Rivera, C. ``Problems & Puzzles (Puzzles): Prime-Magical Squares.'' http://www.sci.net.mx/~crivera/puzzles/puzz_004.htm.

Sloane, N. J. A. SequenceA006052/M5482in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.

Suzuki, M. ``Magic Squares.'' http://www.pse.che.tohoku.ac.jp/~msuzuki/MagicSquare.html.