| 释义 |
Mertens ConjectureGiven Mertens Function defined by
 | (1) |
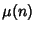 is the Möbius Function, Mertens (1897) conjecture states that is the Möbius Function, Mertens (1897) conjecture states that
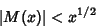 | (2) |
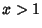 . The conjecture has important implications, since the truth of any equality of the form . The conjecture has important implications, since the truth of any equality of the form
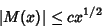 | (3) |
 (the form of Mertens conjecture with (the form of Mertens conjecture with 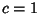 ) would imply the Riemann Hypothesis. In 1885, Stieltjesclaimed that he had a proof that ) would imply the Riemann Hypothesis. In 1885, Stieltjesclaimed that he had a proof that 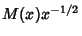 always stayed between two fixed bounds. However, it seems likely thatStieltjes was mistaken. always stayed between two fixed bounds. However, it seems likely thatStieltjes was mistaken.
Mertens conjecture was proved false by Odlyzko and te Riele (1985). Their proof is indirect and does not produce a specificcounterexample, but it does show that
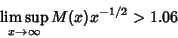 | (4) |
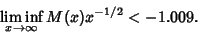 | (5) |
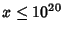 , or even , or even . Pintz (1987) subsequently showed that at least one counterexample to the conjecture occurs for . Pintz (1987) subsequently showed that at least one counterexample to the conjecture occurs for 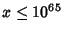 ,using a weighted integral average of ,using a weighted integral average of 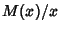 and a discrete sum involving nontrivial zeros of the Riemann ZetaFunction. and a discrete sum involving nontrivial zeros of the Riemann ZetaFunction.
It is still not known if
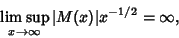 | (6) |
References
Anderson, R. J. ``On the Mertens Conjecture for Cusp Forms.'' Mathematika 26, 236-249, 1979.Anderson, R. J. ``Corrigendum: `On the Mertens Conjecture for Cusp Forms.''' Mathematika 27, 261, 1980. Devlin, K. ``The Mertens Conjecture.'' Irish Math. Soc. Bull. 17, 29-43, 1986. Grupp, F. ``On the Mertens Conjecture for Cusp Forms.'' Mathematika 29, 213-226, 1982. Jurkat, W. and Peyerimhoff, A. ``A Constructive Approach to Kronecker Approximation and Its Application to the Mertens Conjecture.'' J. reine angew. Math. 286/287, 322-340, 1976. Mertens, F. ``Über eine zahlentheoretische Funktion.'' Sitzungsber. Akad. Wiss. Wien IIa 106, 761-830, 1897. Odlyzko, A. M. and te Riele, H. J. J. ``Disproof of the Mertens Conjecture.'' J. reine angew. Math. 357, 138-160, 1985. Pintz, J. ``An Effective Disproof of the Mertens Conjecture.'' Astérique 147-148, 325-333 and 346, 1987. te Riele, H. J. J. ``Some Historical and Other Notes About the Mertens Conjecture and Its Recent Disproof.'' Nieuw Arch. Wisk. 3, 237-243, 1985.
|
