E
ULER
(1707–83) as a device to analyze
ARGUMENT
s, it
was not until the publication of Venn’s 1881 book
Symbolic Logic that the practice of using such dia-
grams became common. Today these diagrams are
named in his honor.
Venn graduated from Gonville and Caius College,
Cambridge, in 1857 after studying theology and the
liberal arts. After working as an ordained priest for
several years, Venn returned to the same institution in
1862 to accept a position as a lecturer in moral science.
During this time he pursued interests in logic and
PROB
-
ABILITY
theory, and developed a “frequency theory” of
probability, which he published in 1866 in his Logic of
Chance. This work greatly influenced the development
of the theory of statistics. Fifteen years later Venn pub-
lished Symbolic Logic, which he followed with The
Principles of Empirical Logic in 1889. Venn was
elected a fellow of the R
OYAL
S
OCIETY
in 1883.
After the publication of his work on logic, Venn
changed interests and took to researching and writing a
comprehensive account of the history of Gonville and
Caius College. This was an all-consuming task, and
only one volume of the work was published before his
death, April 4, 1923.
Venn diagrams have had a profound effect on
modern mathematics education. They are often used as
a device for encouraging logical thinking at the early
stage of a child’s intellectual development and are a
standard feature in an elementary-school curriculum.
See also V
ENN DIAGRAM
.
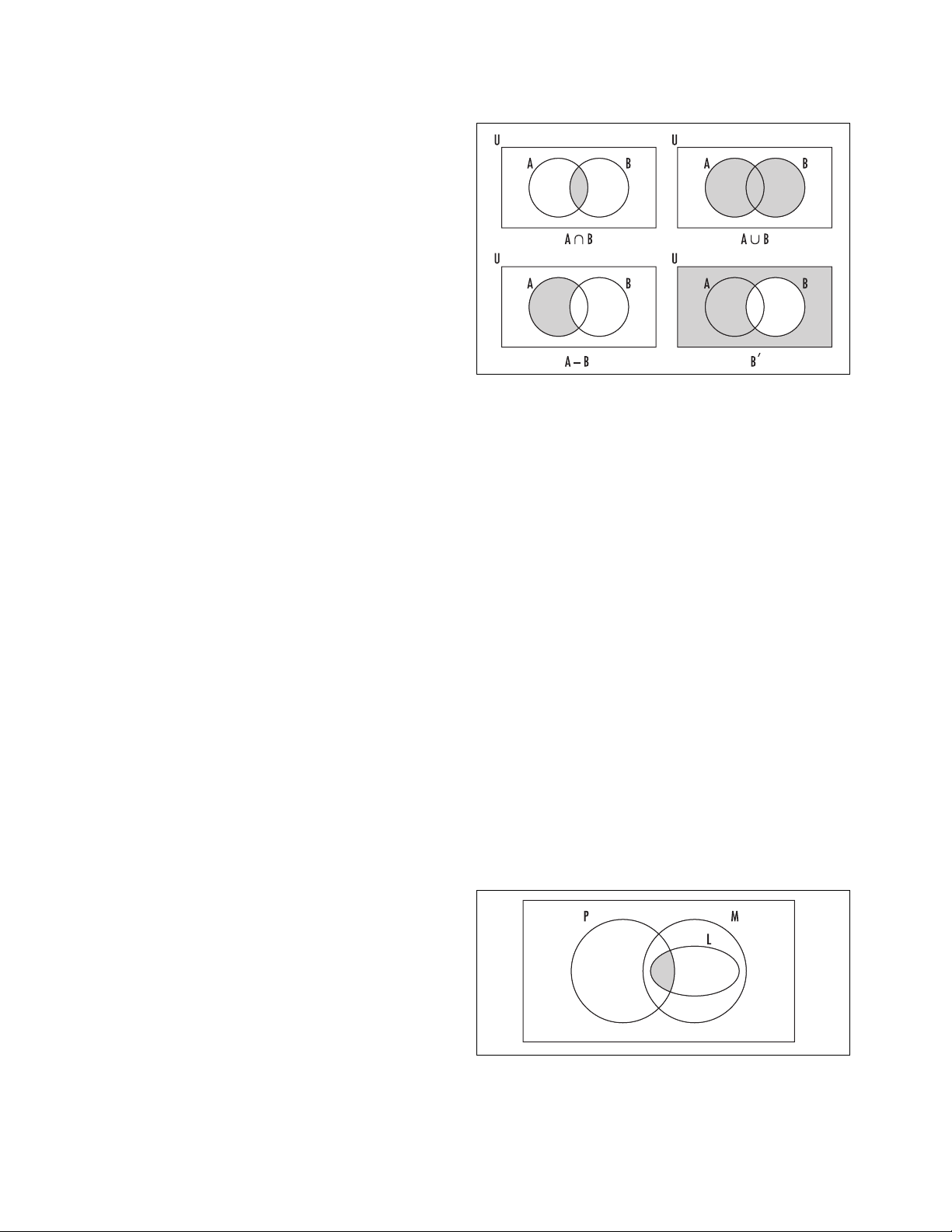
Venn diagram A diagram in which mathematical
sets are represented by overlapping circles within a
boundary representing the universal set is called a Venn
diagram. Such diagrams provide convenient pictorial
representations of relations between sets. For example,
in the diagram above right, a universal set Uis repre-
sented by the interior of a rectangle, and two subsets A
and Bof Uas the interiors of two overlapping circles
within the rectangle. The intersection A∩B, the union
A∪B, the complement of Bwithin A, denoted A– B,
and the universal complement of B, denoted B′, for
instance, are readily apparent.
For example, if Uis the set of all insects, Ais the
subset of all butterflies, and Bis the subset of all blue
insects, then the shaded region A∩Brepresents all
blue butterflies, A∪Ball insects that are either blue or
are a butterfly, A– Ball butterflies that are not blue,
and B′all insects that are not blue.
It is possible to demonstrate the validity of an
ARGUMENT
with the aid of a Venn diagram. Consider,
for example, the following line of reasoning:
All logicians are mathematicians. Some philoso-
phers are logicians. Therefore, some philoso-
phers are mathematicians.
In the universal set of all people, let Lbe the set of
logicians, Mthe set of mathematicians, and Pthe set of
philosophers. The first premise of the argument asserts
that Lis a subset of M, and the second that the sets P
and Lhave a nonempty intersection. This leads to a
Venn diagram of the form below.
It is now apparent that Pand Lcan intersect only
inside of M thereby establishing the validity of the
conclusion. The German mathematician G
OTTFRIED
524 Venn diagram
Set operations
A Venn diagram