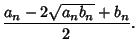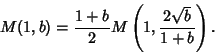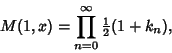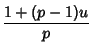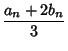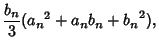| 释义 |
Arithmetic-Geometric MeanThe arithmetic-geometric mean (AGM) 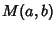 of two numbers of two numbers 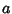 and and 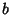 is defined by starting with is defined by starting with 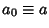 and and 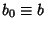 , then iterating , then iterating
until 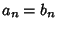 . . 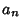 and and 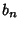 converge towards each other since converge towards each other since
But 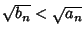 , so , so
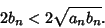 | (4) |
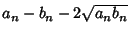 to each side to each side
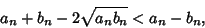 | (5) |
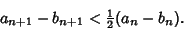 | (6) |
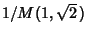 is called Gauss's Constant. is called Gauss's Constant.
The AGM has the properties
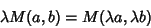 | (7) |
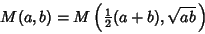 | (8) |
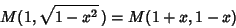 | (9) |
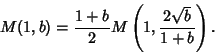 | (10) |
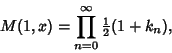 | (11) |
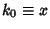 and and
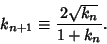 | (12) |
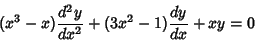 | (13) |
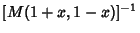 and and 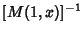 . .
A generalization of the Arithmetic-Geometric Mean is
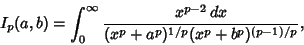 | (14) |
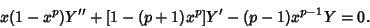 | (15) |
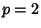 or or 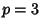 , there is a modular transformation for the solutions of (15) that are bounded as , there is a modular transformation for the solutions of (15) that are bounded as 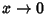 .Letting .Letting 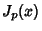 be one of these solutions, the transformation takes the form be one of these solutions, the transformation takes the form
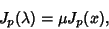 | (16) |
and
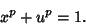 | (19) |
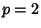 gives the Arithmetic-Geometric Mean, and gives the Arithmetic-Geometric Mean, and 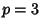 gives a cubic relative discussed by Borwein andBorwein (1990, 1991) and Borwein (1996) in which, for gives a cubic relative discussed by Borwein andBorwein (1990, 1991) and Borwein (1996) in which, for  and and 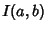 defined by defined by
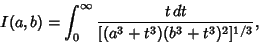 | (20) |
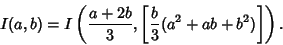 | (21) |
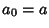 and and 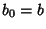 and and
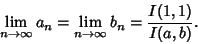 | (24) |
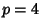 and and 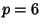 , but they do not give identities for , but they do not give identities for 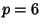 (Borwein 1996).See also Arithmetic-Harmonic Mean (Borwein 1996).See also Arithmetic-Harmonic Mean
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``The Process of the Arithmetic-Geometric Mean.'' §17.6 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 571 ad 598-599, 1972.Borwein, J. M. Problem 10281. ``A Cubic Relative of the AGM.'' Amer. Math. Monthly 103, 181-183, 1996. Borwein, J. M. and Borwein, P. B. ``A Remarkable Cubic Iteration.'' In Computational Method & Function Theory: Proc. Conference Held in Valparaiso, Chile, March 13-18, 1989 (Ed. A. Dold, B. Eckmann, F. Takens, E. B Saff, S. Ruscheweyh, L. C. Salinas, L. C., and R. S. Varga). New York: Springer-Verlag, 1990. Borwein, J. M. and Borwein, P. B. ``A Cubic Counterpart of Jacobi's Identity and the AGM.'' Trans. Amer. Math. Soc. 323, 691-701, 1991. Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 906-907, 1992. |
![]() of two numbers
of two numbers ![]() and
and ![]() is defined by starting with
is defined by starting with ![]() and
and ![]() , then iterating
, then iterating