“Spirograph”的概念、定义、习题解题方法及翻译-数学辞典

单词

Spirograph

释义

Spirograph

A Hypotrochoid generated by a fixed point on a Circle rolling inside a fixed Circle. It has parametricequations,

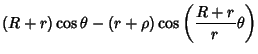			(1)
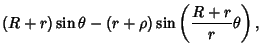			(2)

where

is the radius of the fixed circle,

is the radius of the rotating circle, and

is the offset of the edge ofthe rotating circle. The figure closes only if

, and

are Rational. The equationscan also be written

where the outer wheel has radius 1, the inner wheel a radius

, the pen is placed

units from the center, the beginningis at

radians above the

-axis, and

The following curves are for

, with

, 2, ..., 10, and

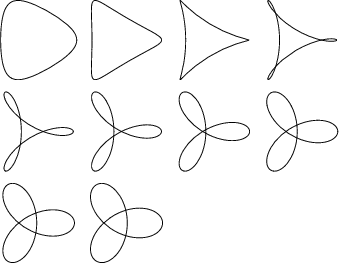
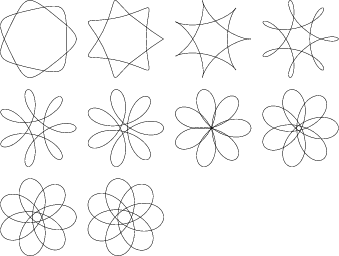
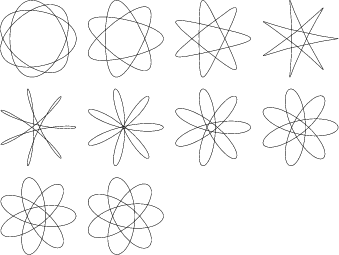
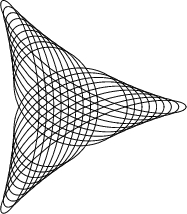
Additional attractive designs such as the following can also be made by superposing individual spirographs.

See also Epitrochoid, Hypotrochoid, Maurer Rose, Spirolateral

随便看

数学辞典收录了8975条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。