| 释义 |
Chebyshev Polynomial of the First KindA set of Orthogonal Polynomials defined as the solutions to the Chebyshev Differential Equation and denoted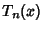 . They are used as an approximation to a Least Squares Fit, and are a specialcase of the Ultraspherical Polynomial with . They are used as an approximation to a Least Squares Fit, and are a specialcase of the Ultraspherical Polynomial with 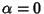 . The Chebyshev polynomials of the first kind . The Chebyshev polynomials of the first kind 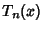 areillustrated above for areillustrated above for 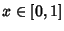 and and 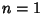 , 2, ..., 5. , 2, ..., 5.
The Chebyshev polynomials of the first kind can be obtained from the generating functions
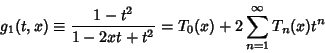 | (1) |
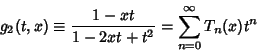 | (2) |
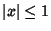 and and  (Beeler et al. 1972, Item 15). (A closely related Generating Function is the basisfor the definition of Chebyshev Polynomial of the Second Kind.) They are normalized such that (Beeler et al. 1972, Item 15). (A closely related Generating Function is the basisfor the definition of Chebyshev Polynomial of the Second Kind.) They are normalized such that 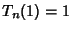 . Theycan also be written . Theycan also be written
 | (3) |
 | (4) |
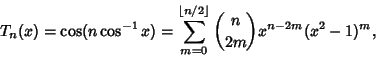 | (5) |
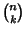 is a Binomial Coefficient and is a Binomial Coefficient and  is the Floor Function.Therefore, zeros occur when is the Floor Function.Therefore, zeros occur when
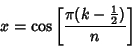 | (6) |
 , 2, ..., , 2, ..., 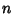 . Extrema occur for . Extrema occur for
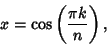 | (7) |
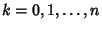 . At maximum, . At maximum,  , and at minimum, , and at minimum, 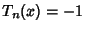 . The Chebyshev Polynomials areOrthonormal with respect to the Weighting Function . The Chebyshev Polynomials areOrthonormal with respect to the Weighting Function 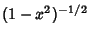
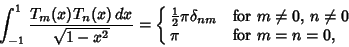 | (8) |
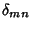 is the Kronecker Delta.Chebyshev polynomials of the first kind satisfy the additional discrete identity is the Kronecker Delta.Chebyshev polynomials of the first kind satisfy the additional discrete identity
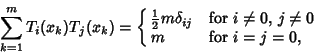 | (9) |
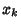 for for  , ..., , ...,  are the are the  zeros of zeros of 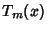 . They also satisfy the RecurrenceRelations . They also satisfy the RecurrenceRelations
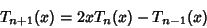 | (10) |
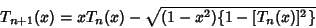 | (11) |
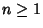 . They have a Complex integral representation . They have a Complex integral representation
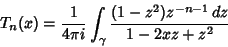 | (12) |
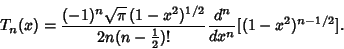 | (13) |
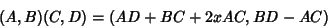 | (14) |
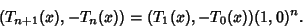 | (15) |
Using Gram-Schmidt Orthonormalization in the range ( ,1) with Weighting Function ,1) with Weighting Function 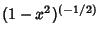 gives gives
etc. Normalizing such that 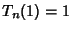 gives gives
The Chebyshev polynomial of the first kind is related to the Bessel Function of the First Kind 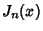 andModified Bessel Function of the First Kind andModified Bessel Function of the First Kind 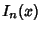 by the relations by the relations
 | (19) |
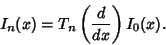 | (20) |
Letting  allows the Chebyshev polynomials of the first kind to be written as allows the Chebyshev polynomials of the first kind to be written as
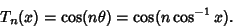 | (21) |
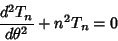 | (22) |
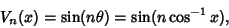 | (23) |
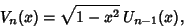 | (24) |
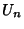 is a Chebyshev Polynomial of the Second Kind. Note that is a Chebyshev Polynomial of the Second Kind. Note that 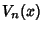 is therefore not a Polynomial. is therefore not a Polynomial.
The Polynomial
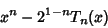 | (25) |
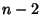 ) is the Polynomial of degree ) is the Polynomial of degree 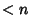 which stays closest to which stays closest to  in the interval in the interval 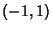 . The maximumdeviation is . The maximumdeviation is 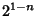 at the at the 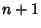 points where points where
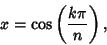 | (26) |
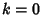 , 1, ..., , 1, ..., 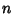 (Beeler et al. 1972, Item 15).See also Chebyshev Approximation Formula, Chebyshev Polynomial of the Second Kind (Beeler et al. 1972, Item 15).See also Chebyshev Approximation Formula, Chebyshev Polynomial of the Second Kind
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Orthogonal Polynomials.'' Ch. 22 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 771-802, 1972.Arfken, G. ``Chebyshev (Tschebyscheff) Polynomials'' and ``Chebyshev Polynomials--Numerical Applications.'' §13.3 and 13.4 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 731-748, 1985. Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972. Iyanaga, S. and Kawada, Y. (Eds.). ``Cebysev (Tschebyscheff) Polynomials.'' Appendix A, Table 20.II in Encyclopedic Dictionary of Mathematics. Cambridge, MA: MIT Press, pp. 1478-1479, 1980. Rivlin, T. J. Chebyshev Polynomials. New York: Wiley, 1990. Spanier, J. and Oldham, K. B. ``The Chebyshev Polynomials 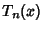 and and  .'' Ch. 22 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 193-207, 1987. .'' Ch. 22 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 193-207, 1987.
|
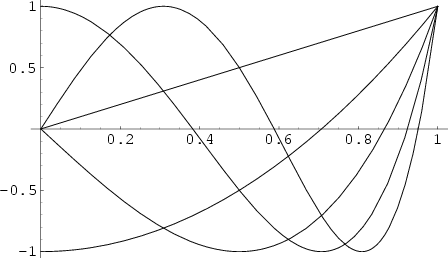
![]() . They are used as an approximation to a Least Squares Fit, and are a specialcase of the Ultraspherical Polynomial with
. They are used as an approximation to a Least Squares Fit, and are a specialcase of the Ultraspherical Polynomial with ![]() . The Chebyshev polynomials of the first kind
. The Chebyshev polynomials of the first kind ![]() areillustrated above for
areillustrated above for ![]() and
and ![]() , 2, ..., 5.
, 2, ..., 5.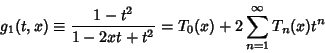
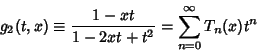


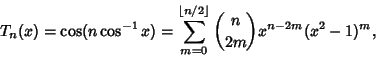
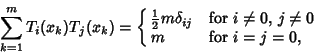
![]() ,1) with Weighting Function
,1) with Weighting Function ![]() gives
gives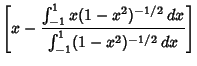
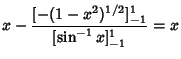
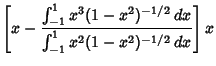
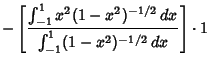
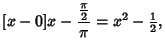
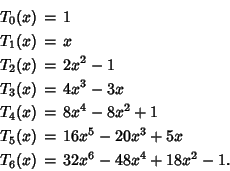
![]() andModified Bessel Function of the First Kind
andModified Bessel Function of the First Kind ![]() by the relations
by the relations![]() allows the Chebyshev polynomials of the first kind to be written as
allows the Chebyshev polynomials of the first kind to be written as![]() and
and ![]() .'' Ch. 22 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 193-207, 1987.
.'' Ch. 22 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 193-207, 1987.