Triangle
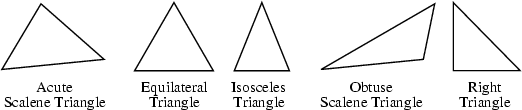
A triangle is a 3-sided Polygon sometimes (but not very commonly) called the Trigon. All triangles areconvex. An Acute Triangle is a triangle whose three angles are all Acute. A triangle withall sides equal is called Equilateral. A triangle with two sides equal is calledIsosceles. A triangle having an Obtuse Angle is called an Obtuse Triangle. Atriangle with a Right Angle is called Right. A triangle with all sides a different lengthis called Scalene.
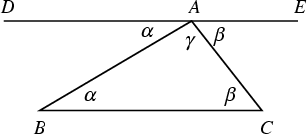
The sum of Angles in a triangle is 180°. This can be established as follows. Let ![]() (
(![]() be Parallel to
be Parallel to ![]() ) in the above diagram, then the angles
) in the above diagram, then the angles ![]() and
and ![]() satisfy
satisfy ![]() and
and ![]() , as indicated. Adding
, as indicated. Adding ![]() , it follows that
, it follows that
| (1) |
Let ![]() stand for a triangle side and
stand for a triangle side and ![]() for an angle, and let a set of
for an angle, and let a set of ![]() s and
s and ![]() s be concatenated such that adjacent letterscorrespond to adjacent sides and angles in a triangle. Triangles are uniquely determined by specifying three sides(SSS Theorem), two angles and a side (AAS Theorem), or two sides with an adjacent angle (SAS Theorem).In each of these cases, the unknown three quantities (there are three sides and three angles total) can be uniquelydetermined. Other combinations of sides and angles do not uniquely determine a triangle: three angles specify atriangle only modulo a scale size (AAA Theorem), and one angle and two sides not containing it may specify one,two, or no triangles (ASS Theorem).
s be concatenated such that adjacent letterscorrespond to adjacent sides and angles in a triangle. Triangles are uniquely determined by specifying three sides(SSS Theorem), two angles and a side (AAS Theorem), or two sides with an adjacent angle (SAS Theorem).In each of these cases, the unknown three quantities (there are three sides and three angles total) can be uniquelydetermined. Other combinations of sides and angles do not uniquely determine a triangle: three angles specify atriangle only modulo a scale size (AAA Theorem), and one angle and two sides not containing it may specify one,two, or no triangles (ASS Theorem).
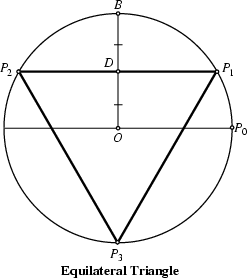
The Straightedge and Compass construction of the triangle can be accomplished as follows. In the above figure, take![]() as a Radius and draw
as a Radius and draw ![]() . Then bisect
. Then bisect ![]() and construct
and construct ![]() . Extending
. Extending ![]() tolocate
tolocate ![]() then gives the Equilateral Triangle
then gives the Equilateral Triangle ![]() .
.
In Proposition IV.4 of the Euclid ![]() showed how to inscribe a Circle (theIncircle) in a given triangle by locating the Center as the point of intersection of AngleBisectors. In Proposition IV.5, he showed how to circumscribe a Circle (the Circumcircle)about a given triangle by locating the Center as the point of intersection of the perpendicular bisectors.
showed how to inscribe a Circle (theIncircle) in a given triangle by locating the Center as the point of intersection of AngleBisectors. In Proposition IV.5, he showed how to circumscribe a Circle (the Circumcircle)about a given triangle by locating the Center as the point of intersection of the perpendicular bisectors.
If the coordinates of the triangle Vertices are given by ![]() where
where ![]() 2, 3, thenthe Area
2, 3, thenthe Area ![]() is given by the Determinant
is given by the Determinant
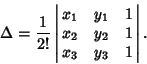 | (2) |
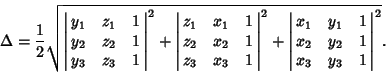 | (3) |
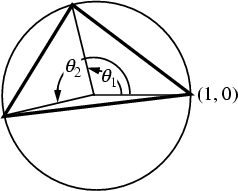
In the above figure, let the Circumcircle passing through a triangle's Vertices haveRadius ![]() , and denote the Central Angles from the first point to the second
, and denote the Central Angles from the first point to the second ![]() ,and to the third point by
,and to the third point by ![]() . Then the Area of the triangle is given by
. Then the Area of the triangle is given by
| (4) |
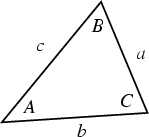
If a triangle has sides ![]() ,
, ![]() ,
, ![]() , call the angles opposite these sides
, call the angles opposite these sides ![]() ,
, ![]() , and
, and ![]() , respectively. Alsodefine the Semiperimeter
, respectively. Alsodefine the Semiperimeter ![]() as Half the Perimeter:
as Half the Perimeter:
| (5) |
| (6) |
| (7) | |||
| (8) | |||
| (9) | |||
| (10) | |||
| (11) | |||
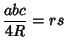 | (12) | ||
| (13) | |||
| (14) |
In the above formulas,
| (15) | |||
| (16) | |||
| (17) |
gives the particularly pretty form
| (18) |
The Angles of a triangle satisfy
| (19) |
| (20) |
Let a triangle have Angles ![]() ,
, ![]() , and
, and ![]() . Then
. Then
| (21) |
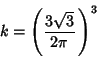 | (22) |
| (23) |
Trigonometric Functions of half angles can be expressed in terms of the triangle sides:
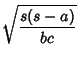 | (24) | ||
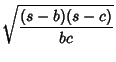 | (25) | ||
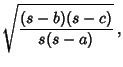 | (26) |
where
The number of different triangles which have Integral sides and Perimeter ![]() is
is
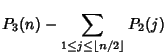 | |||
 | |||
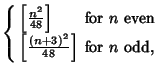 | (27) |
where
| (28) |
It is not known if a triangle with Integer sides, Medians, and Area exists(although there are incorrect Proofs of the impossibility in the literature). However, R. L. Rathbun,A. Kemnitz, and R. H. Buchholz have shown that there are infinitely many triangles with Rational sides (Heronian Triangles) with two Rational Medians (Guy 1994).
In the following paragraph, assume the specified sides and angles are adjacent to each other. Specifying threeAngles does not uniquely define a triangle, but any two triangles with the same Anglesare similar (the AAA Theorem). Specifying two Angles ![]() and
and ![]() and a side
and a side ![]() uniquely determines a triangle with Area
uniquely determines a triangle with Area
| (29) |
| (30) |
| (31) |
| (32) |
There are four Circles which are tangent to the sides of a triangle, one internal and the rest external.Their centers are the points of intersection of the Angle Bisectors of the triangle.
Any triangle can be positioned such that its shadow under an orthogonal projection is Equilateral.
See also AAA Theorem, AAS Theorem, Acute Triangle, Alcuin's Sequence, Altitude, AngleBisector, Anticevian Triangle, Anticomplementary Triangle, Antipedal Triangle, ASS Theorem,Bell Triangle, Brianchon Point, Brocard Angle, Brocard Circle, Brocard Midpoint,Brocard Points, Butterfly Theorem, Centroid (Triangle), Ceva's Theorem, Cevian,Cevian Triangle, Chasles's Theorem, Circumcenter, Circumcircle, Circumradius,Contact Triangle, Crossed Ladders Problem, Crucial Point, D-Triangle, de LongchampsPoint, Desargues' Theorem, Dissection, Elkies Point, Equal Detour Point, EquilateralTriangle, Euler Line, Euler's Triangle, Euler Triangle Formula, Excenter, ExcentralTriangle, Excircle, Exeter Point, Exmedian, Exmedian Point, Exradius, ExteriorAngle Theorem, Fagnano's Problem, Far-Out Point, Fermat Point, Fermat's Problem,Feuerbach Point, Feuerbach's Theorem, Fuhrmann Triangle, Gergonne Point, Grebe Point,Griffiths Points, Griffiths' Theorem, Harmonic Conjugate Points, Heilbronn Triangle Problem,Heron's Formula, Heronian Triangle, Hofstadter Triangle, Homothetic Triangles, Incenter,Incircle, Inradius, Isodynamic Points, Isogonal Conjugate, Isogonic Centers,Isoperimetric Point, Isosceles Triangle, Kabon Triangles, Kanizsa Triangle, Kiepert'sHyperbola, Kiepert's Parabola, Law of Cosines, Law of Sines, Law of Tangents, LeibnizHarmonic Triangle, Lemoine Circle, Lemoine Point, Line at Infinity, Malfatti Points,Medial Triangle, Median (Triangle), Median Triangle, Menelaus' Theorem, Mid-Arc Points,Mittenpunkt, Mollweide's Formulas, Morley Centers, Morley's Theorem, Nagel Point,Napoleon's Theorem, Napoleon Triangles, Newton's Formulas, Nine-Point Circle, NumberTriangle, Obtuse Triangle, Orthic Triangle, Orthocenter, Orthologic, ParalogicTriangles, Pascal's Triangle, Pasch's Axiom, Pedal Triangle, Perpendicular Bisector,Perspective Triangles, Petersen-Shoute Theorem, Pivot Theorem, Power Point, Power(Triangle), Prime Triangle, Purser's Theorem, Quadrilateral, Rational Triangle, Routh'sTheorem, SAS Theorem, Scalene Triangle, Schiffler Point, Schwarz Triangle, Schwarz'sTriangle Problem, Seidel-Entringer-Arnold Triangle, Seydewitz's Theorem, Simson Line, SpiekerCenter, SSS Theorem, Steiner-Lehmus Theorem, Steiner Points, Stewart's Theorem,Symmedian Point, Tangential Triangle, Tangential Triangle Circumcenter, Tarry Point,Thomsen's Figure, Torricelli Point, Triangle Tiling, Triangle Transformation Principle,Yff Points, Yff TrianglesReferences
Abi-Khuzam, F. ``Proof of Yff's Conjecture on the Brocard Angle of a Triangle.'' Elem. Math. 29, 141-142, 1974. Andrews, G. ``A Note on Partitions and Triangles with Integer Sides.'' Amer. Math. Monthly 86, 477, 1979. Baker, M. ``A Collection of Formulæ for the Area of a Plane Triangle.'' Ann. Math. 1, 134-138, 1884. Berkhan, G. and Meyer, W. F. ``Neuere Dreiecksgeometrie.'' In Encyklopaedie der Mathematischen Wissenschaften, Vol. 3AB 10 (Ed. F. Klein). Leipzig: Teubner, pp. 1173-1276, 1914. Beyer, W. H. (Ed.) CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 123-124, 1987. Coxeter, H. S. M. Introduction to Geometry, 2nd ed. New York: Wiley, 1969. Davis, P. ``The Rise, Fall, and Possible Transfiguration of Triangle Geometry: A Mini-History.'' Amer. Math. Monthly 102, 204-214, 1995. Eppstein, D. ``Triangles and Simplices.''http://www.ics.uci.edu/~eppstein/junkyard/triangulation.html. Feuerbach, K. W. Eigenschaften einiger merkwürdingen Punkte des geradlinigen Dreiecks, und mehrerer durch die bestimmten Linien und Figuren. Nürnberg, Germany, 1822. Guy, R. K. ``Triangles with Integer Sides, Medians, and Area.'' §D21 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 188-190, 1994. Honsberger, R. Mathematical Gems III. Washington, DC: Math. Assoc. Amer., pp. 39-47, 1985. Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, 1929. Jordan, J. H.; Walch, R.; and Wisner, R. J. ``Triangles with Integer Sides.'' Amer. Math. Monthly 86, 686-689, 1979. Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994. Kimberling, C. ``Triangle Centers and Central Triangles.'' Congr. Numer. 129, 1-295, 1998. Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 28, 1983. Schroeder. Das Dreieck und seine Beruhungskreise. Sloane, N. J. A. SequenceA005044/M0146in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995. Vandeghen, A. ``Some Remarks on the Isogonal and Cevian Transforms. Alignments of Remarkable Points of a Triangle.'' Amer. Math. Monthly 72, 1091-1094, 1965.![]() Weisstein, E. W. ``Plane Geometry.'' Mathematica notebook PlaneGeometry.m.
Weisstein, E. W. ``Plane Geometry.'' Mathematica notebook PlaneGeometry.m.
- Condition Number
- Condom Problem
- Condon-Shortley Phase
- Conductor
- Cone
- Cone Graph
- Cone Net
- Cone (Space)
- Cone-Sphere Intersection
- Confidence Interval
- Configuration
- Confluent Hypergeometric Differential Equation
- Confluent Hypergeometric Function
- Confluent Hypergeometric Function of the First Kind
- Confluent Hypergeometric Function of the Second Kind
- Confluent Hypergeometric Limit Function
- Confocal Conics
- Confocal Ellipsoidal Coordinates
- Confocal Parabolic Coordinates
- Confocal Paraboloidal Coordinates
- Conformal Latitude
- Conformal Map
- Conformal Solution
- Conformal Tensor
- Conformal Transformation