| 释义 |
k-StatisticAn Unbiased Estimator of the Cumulants 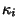 of a Distribution. The expectation values of the of a Distribution. The expectation values of the  -statistics are therefore given by the correspondingCumulants -statistics are therefore given by the correspondingCumulants
(Kenney and Keeping 1951, p. 189). For a sample of size, 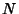 , the first few , the first few  -statistics are given by -statistics are given by
where 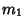 is the sample Mean, is the sample Mean, 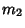 is the sample Variance, and is the sample Variance, and 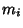 is the sample is the sample  th Moment about theMean (Kenney and Keeping 1951, pp. 109-110, 163-165, and 189; Kenney and Keeping 1962). These statistics are obtainedfrom inverting the relationships th Moment about theMean (Kenney and Keeping 1951, pp. 109-110, 163-165, and 189; Kenney and Keeping 1962). These statistics are obtainedfrom inverting the relationships
The first moment (sample Mean) is
 | (14) |
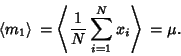 | (15) |
and the expectation value is
since there are 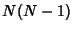 terms terms 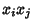 , using , using
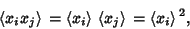 | (18) |
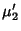 is the Moment about 0. Using the identity is the Moment about 0. Using the identity
 | (19) |
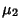 about the Mean and simplifying then gives about the Mean and simplifying then gives
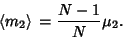 | (20) |
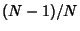 is known as Bessel's Correction. is known as Bessel's Correction.
The third Moment is
Now use the identities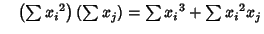 | (22) | 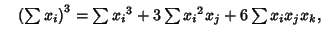 | (23) |
where it is understood that sums over products of variables exclude equal indices. Plugging in
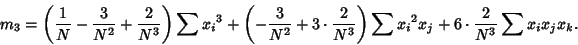 | (24) |
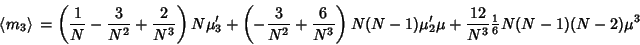 | (25) |
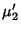 is the Moment about 0. Plugging in the identities is the Moment about 0. Plugging in the identities
and simplifying then gives
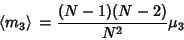 | (28) |
The fourth Moment is
Now use the identitiesPlugging in,
The expectation value is then given by
where 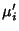 are Moments about 0. Using the identities are Moments about 0. Using the identities
and simplifying gives
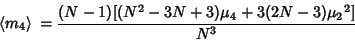 | (38) |
The square of the second moment is
Now use the identitiesPlugging in,
The expectation value is then given by
where 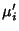 are Moments about 0. Using the identities are Moments about 0. Using the identities
and simplifying gives
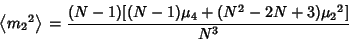 | (48) |
The Variance of 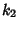 is given by is given by
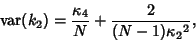 | (49) |
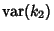 is given by is given by
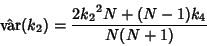 | (50) |
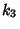 can be expressed in terms of Cumulants by can be expressed in terms of Cumulants by
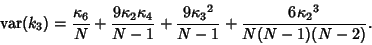 | (51) |
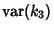 is is
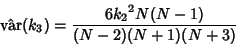 | (52) |
Now consider a finite population. Let a sample of 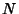 be taken from a population of be taken from a population of 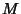 . Then UnbiasedEstimators . Then UnbiasedEstimators  for the population Mean for the population Mean 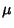 , ,  for the population Variance for the population Variance 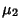 , ,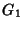 for the population Skewness for the population Skewness 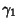 , and , and 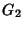 for the population Kurtosis for the population Kurtosis 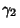 are are
(Church 1926, p. 357; Carver 1930; Irwin and Kendall 1944; Kenney and Keeping 1951, p. 143), where 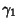 is the sampleSkewness and is the sampleSkewness and 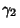 is the sample Kurtosis.See also Gaussian Distribution, Kurtosis, Mean, Moment, Skewness, Variance is the sample Kurtosis.See also Gaussian Distribution, Kurtosis, Mean, Moment, Skewness, Variance
References
Carver, H. C. (Ed.). ``Fundamentals of the Theory of Sampling.'' Ann. Math. Stat. 1, 101-121, 1930.Church, A. E. R. ``On the Means and Squared Standard-Deviations of Small Samples from Any Population.'' Biometrika 18, 321-394, 1926. Irwin, J. O. and Kendall, M. G. ``Sampling Moments of Moments for a Finite Population.'' Ann. Eugenics 12, 138-142, 1944. Kenney, J. F. and Keeping, E. S. Mathematics of Statistics, Pt. 2, 2nd ed. Princeton, NJ: Van Nostrand, 1951. Kenney, J. F. and Keeping, E. S. ``The  -Statistics.'' §7.9 in Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, pp. 99-100, 1962. -Statistics.'' §7.9 in Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, pp. 99-100, 1962.
|
![]() of a Distribution. The expectation values of the
of a Distribution. The expectation values of the ![]() -statistics are therefore given by the correspondingCumulants
-statistics are therefore given by the correspondingCumulants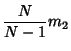
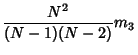
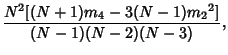
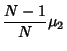
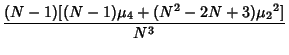
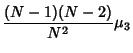
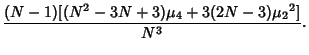

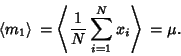
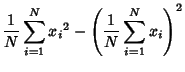
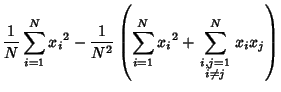
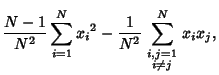
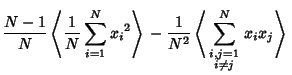
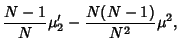
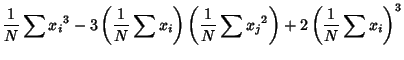
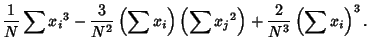

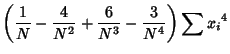
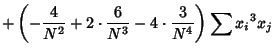
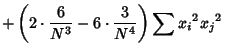
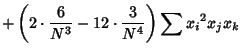
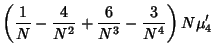
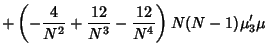
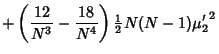
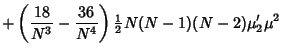
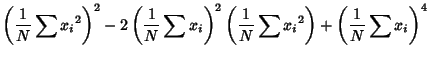
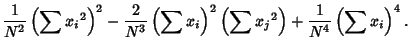
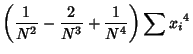
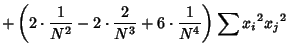
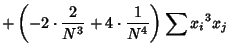
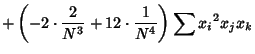
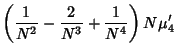
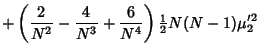
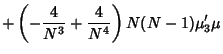
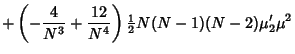
![]() is given by
is given by![]() be taken from a population of
be taken from a population of ![]() . Then UnbiasedEstimators
. Then UnbiasedEstimators ![]() for the population Mean
for the population Mean ![]() ,
, ![]() for the population Variance
for the population Variance ![]() ,
,![]() for the population Skewness
for the population Skewness ![]() , and
, and ![]() for the population Kurtosis
for the population Kurtosis ![]() are
are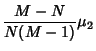
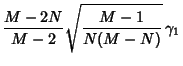
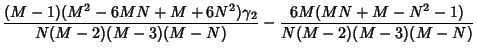
![]() -Statistics.'' §7.9 in Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, pp. 99-100, 1962.
-Statistics.'' §7.9 in Mathematics of Statistics, Pt. 1, 3rd ed. Princeton, NJ: Van Nostrand, pp. 99-100, 1962.