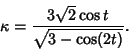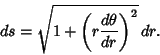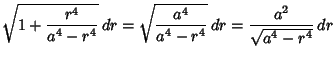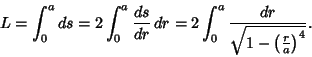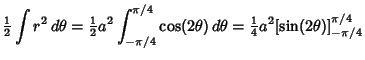| 释义 |
LemniscateA polar curve also called Lemniscate of Bernoulli which is the Locus of points the product of whose distancesfrom two points (called the Foci) is a constant. Letting the Foci be located at 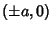 , the Cartesian equation is , the Cartesian equation is
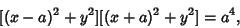 | (1) |
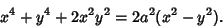 | (2) |
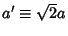 , the Polar Coordinates are given by , the Polar Coordinates are given by
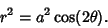 | (3) |
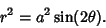 | (4) |
The bipolar equation of the lemniscate is
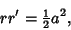 | (7) |
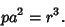 | (8) |
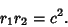 | (9) |
Jakob Bernoulli  published an article in Acta Eruditorum in 1694 in which he calledthis curve the lemniscus (``a pendant ribbon''). Jakob Bernoulli was not aware that the curve he was describing was aspecial case of Cassini published an article in Acta Eruditorum in 1694 in which he calledthis curve the lemniscus (``a pendant ribbon''). Jakob Bernoulli was not aware that the curve he was describing was aspecial case of Cassini  in 1680. The general properties ofthe lemniscate were discovered by G. Fagnano in 1750 (MacTutor Archive). Gauss's in 1680. The general properties ofthe lemniscate were discovered by G. Fagnano in 1750 (MacTutor Archive). Gauss's  andEuler's andEuler's  investigations of the Arc Length of the curve led to later work on EllipticFunctions. investigations of the Arc Length of the curve led to later work on EllipticFunctions.
The Curvature of the lemniscate is
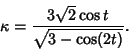 | (10) |
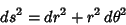 | (11) |
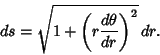 | (12) |
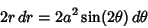 | (13) |
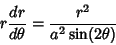 | (14) |
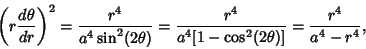 | (15) |
and
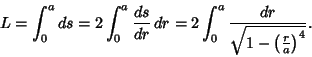 | (17) |
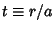 , so , so 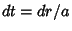 , and , and
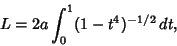 | (18) |
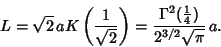 | (19) |
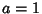 , then , then
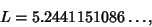 | (20) |
 by by
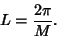 | (21) |
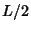 or or 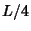 is called the Lemniscate Constant and plays a role for the lemniscate analogous tothat of is called the Lemniscate Constant and plays a role for the lemniscate analogous tothat of 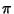 for the Circle. for the Circle.
The Area of one loop of the lemniscate is
See also Lemniscate Function
References
Ayoub, R. ``The Lemniscate and Fagnano's Contributions to Elliptic Integrals.'' Arch. Hist. Exact Sci. 29, 131-149, 1984.Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, 1987. Gray, A. ``Lemniscates of Bernoulli.'' §3.2 in Modern Differential Geometry of Curves and Surfaces. Boca Raton, FL: CRC Press, pp. 39-41, 1993. Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 120-124, 1972. Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 37, 1983. Lee, X. ``Lemniscate of Bernoulli.'' http://www.best.com/~xah/SpecialPlaneCurves_dir/LemniscateOfBernoulli_dir/lemniscateOfBernoulli.html. Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, 1967. MacTutor History of Mathematics Archive. ``Lemniscate of Bernoulli.''http://www-groups.dcs.st-and.ac.uk/~history/Curves/Lemniscate.html. Yates, R. C. ``Lemniscate.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 143-147, 1952.
|
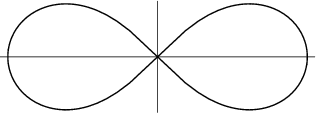
![]() , the Cartesian equation is
, the Cartesian equation is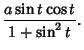
![]() published an article in Acta Eruditorum in 1694 in which he calledthis curve the lemniscus (``a pendant ribbon''). Jakob Bernoulli was not aware that the curve he was describing was aspecial case of Cassini
published an article in Acta Eruditorum in 1694 in which he calledthis curve the lemniscus (``a pendant ribbon''). Jakob Bernoulli was not aware that the curve he was describing was aspecial case of Cassini ![]() in 1680. The general properties ofthe lemniscate were discovered by G. Fagnano in 1750 (MacTutor Archive). Gauss's
in 1680. The general properties ofthe lemniscate were discovered by G. Fagnano in 1750 (MacTutor Archive). Gauss's ![]() andEuler's
andEuler's ![]() investigations of the Arc Length of the curve led to later work on EllipticFunctions.
investigations of the Arc Length of the curve led to later work on EllipticFunctions.