Morley's Theorem
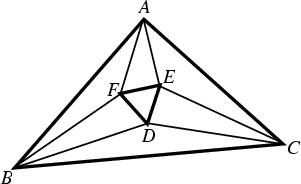
The points of intersection of the adjacent Trisectors of the Angles of anyTriangle 
are the Vertices of an Equilateral Triangle 
known as Morley's Triangle. Taylor and Marr (1914) give two geometric proofs and onetrigonometric proof.
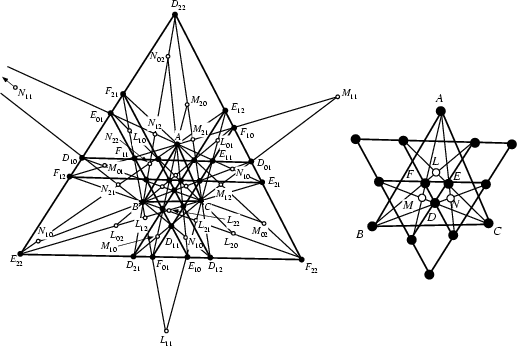
A generalization of Morley's Theorem was discovered by Morley in 1900 but first published by Taylor and Marr (1914).Each Angle of a Triangle has six trisectors, since each interior angle trisector has two associatedlines making angles of 120° with it. The generalization of Morley's theorem states that these trisectors intersect in27 points (denoted 
, 
, 
, for 
, 1, 2) which lie six by six on nine lines. Furthermore, theselines are in three triples of Parallel lines, (
, 
, 
), (
,
, 
), and (
, 
, 
), making Angles of60° with one another (Taylor and Marr 1914, Johnson 1929, p. 254).
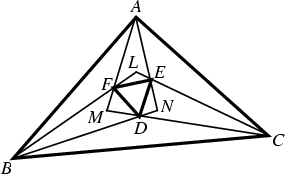
Let 
, 
, and 
be the other trisector-trisector intersections, and let the 27 points 
, 
, 
for, 1, 2 be the Isogonal Conjugates of 
, 
, and 
. Then these points lie 6 by 6 on 9Conics through . In addition, these Conics meet 3 by 3 on theCircumcircle, and the three meeting points form an Equilateral Triangle whose sides are Parallel to thoseof .
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 47-50, 1967. Gardner, M. Martin Gardner's New Mathematical Diversions from Scientific American. New York: Simon and Schuster, pp. 198 and 206, 1966. Honsberger, R. ``Morley's Theorem.'' Ch. 8 in Mathematical Gems I. Washington, DC: Math. Assoc. Amer., pp. 92-98, 1973. Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 253-256, 1929. Kimberling, C. ``Hofstadter Points.'' Nieuw Arch. Wiskunder 12, 109-114, 1994. Marr, W. L. ``Morley's Trisection Theorem: An Extension and Its Relation to the Circles of Apollonius.'' Proc. Edinburgh Math. Soc. 32, 136-150, 1914. Oakley, C. O. and Baker, J. C. ``The Morley Trisector Theorem.'' Amer. Math. Monthly 85, 737-745, 1978. Pappas, T. ``Trisecting & the Equilateral Triangle.'' The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, p. 174, 1989. Taylor, F. G. ``The Relation of Morley's Theorem to the Hessian Axis and Circumcentre.'' Proc. Edinburgh Math. Soc. 32, 132-135, 1914. Taylor, F. G. and Marr, W. L. ``The Six Trisectors of Each of the Angles of a Triangle.'' Proc. Edinburgh Math. Soc. 32, 119-131, 1914.
- Geometric Distribution
- Geometric Mean
- Geometric Mean Index
- Geometric Probability Constants
- Geometric Problems of Antiquity
- Geometric Progression
- Geometric Sequence
- Geometric Series
- Geometrization Conjecture
- Geometrography
- Geometry
- Gergonne Line
- Gergonne Point
- Germain Primes
- Gerono Lemniscate
- Gersgorin Circle Theorem
- G-Function
- g-Function
- Ghost
- Gibbs Constant
- Gibbs Effect
- Gibbs Phenomenon
- Gigantic Prime
- Gilbrat's Distribution
- Gilbreath's Conjecture