inverse matrix 281

1

––

f′(y)

dy

––

dx

√x2– 1

√x2+ 1

√1 + x2

√1 + sinh2y

ey– e–y

––––

2

ey+ e–y

––––

2

provided that the quantity in the denominator is not

zero. This formula can be established by making use of

the

CHAIN RULE

in the statement f(y) = x. (Differentiating

gives: f′(y) · y′= 1 and so y′= , which is the above

formula.)

A simple version of a general inverse-function theo-

rem states that if the derivative of a function y= f(x) is

nonzero at a point x= a, then an inverse function exists,

at least when the domain is restricted to a small interval

about a. (Since the derivative of f(x) = x2is zero at x=

0, it is not possible to define an inverse function to the

squaring function about the point x= 0.)

inverse hyperbolic functions (area hyperbolic func-

tions) Defined in an analogous way to the

INVERSE

TRIGONOMETRIC FUNCTIONS

, the inverse hyperbolic

functions are the inverse functions of the

HYPERBOLIC

FUNCTIONS

. For instance, the inverse hyperbolic sine of

a number x, written arc sinh xor sinh–1x, is a value a

whose hyperbolic sine is x: sinh a= x. Similarly, the

inverse hyperbolic cosine of xis a value awith cosh a=

x, and the inverse hyperbolic tangent of xis a value a

such that tanh a= x. (Technically, for a given value x

there are two different values afor which cosh a= x,

one positive and one negative. By convention, the posi-

tive value is always chosen.)

Since the hyperbolic sine function is defined on all

real values and yields all real values as possible out-

puts, the function sinh–1 xis defined for all real values

of x. On the other hand, the hyperbolic cosine function

only yields output values greater than or equal to 1,

and consequently the inverse hyperbolic cosine func-

tion cosh–1 xis defined only for values of x≥1. Simi-

larly, the inverse hyperbolic tangent function tanh–1 xis

defined only for –1 < x< 1.

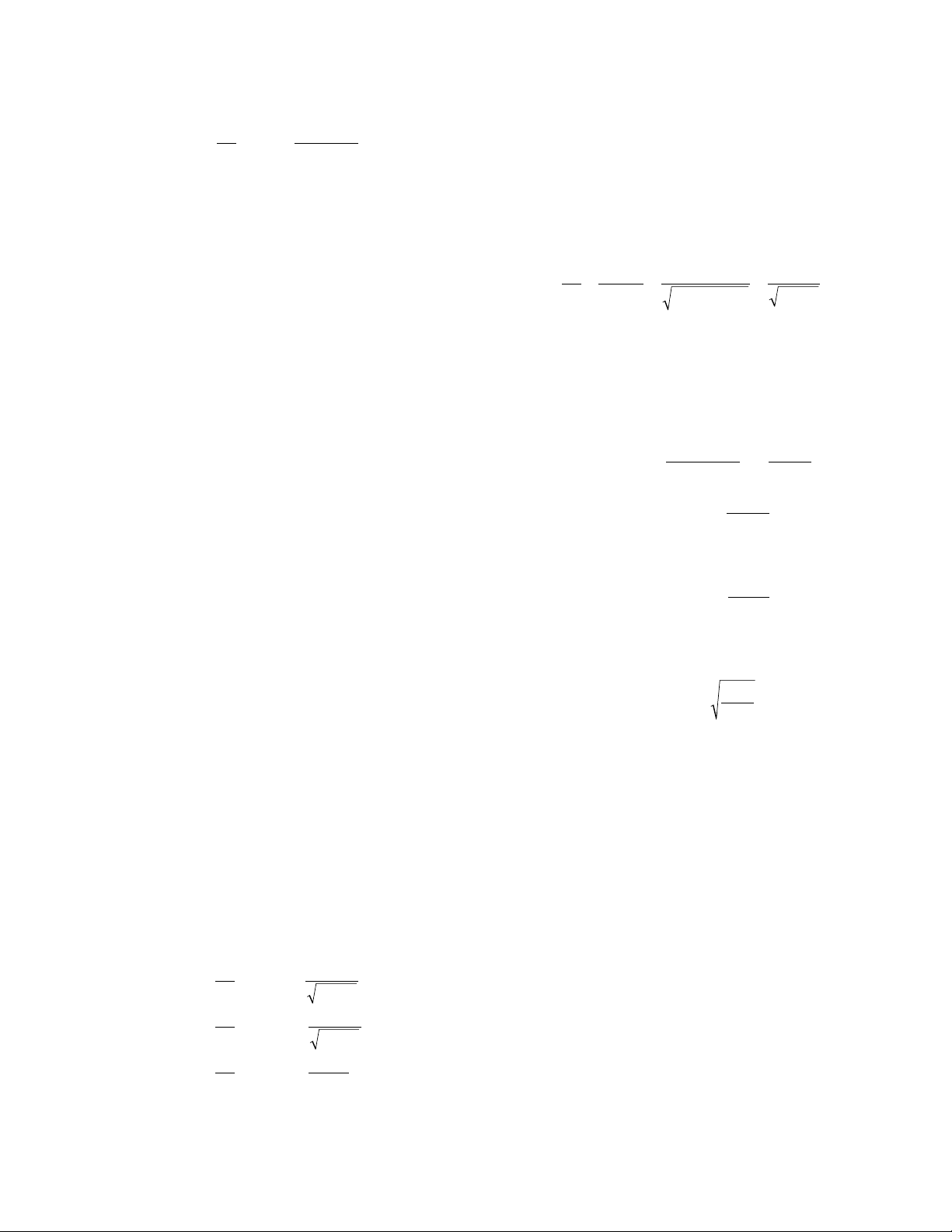
The inverse hyperbolic functions have the follow-

ing

DERIVATIVE

s:

These can be established by making use of the relation

cosh2y– sinh2y= 1. For instance, to compute the

derivative of y= sinh–1 x, write sinh y= xand then dif-

ferentiate this equation making use of the

CHAIN RULE

.

This yields cosh y· = 1, thereby establishing:

as claimed.

It is possible to give alternative formulations of the

inverse hyperbolic functions. Noting that cosh y=

and sinh y= , we have cosh y+ sinh y

= ey or y= ln(cosh y+ sinh y). Set y= sinh–1 x. Then

sinh y= xand cosh y= = , yielding:

sinh–1 x= ln(x+ )

which is valid for all values of x. Similarly,

cosh–1 x= ln(x+ )

valid for x≥1, and

valid for – 1 < x< 1.

inverse matrix (matrix inverse) A square

MATRIX

A

is said to be invertible (or nonsingular) if there is a

matrix Bsuch that AB = BA = I, where Iis the

IDEN

-

TITY MATRIX

. The matrix Bis called the inverse matrix

to A. There is at most one inverse matrix to given

matrix A. (If B1and B2are both inverse matrices, then

B1= IB1= B2AB1= B2I= B2.) If an inverse matrix for a

matrix Aexists, then it is denoted A–1. A study of

DETERMINANT

s shows that a matrix is invertible if, and

only if, its determinant is not zero.

The matrix inverse of a 2 ×2 matrix