polynomial
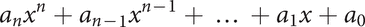
is a polynomial in x with coefficients a0, a1,…, an. When a0, a1,…, an are not all zero, it can be assumed that an ≠ 0 and the polynomial has degree n. For example,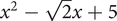 
and 
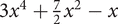are polynomials of degrees 2 and 4 respectively. A polynomial can be denoted by f(x) (so that f is a function), and then f(−1), for example, denotes the value of the polynomial when x is replaced by −1. In the same way, it is possible to consider polynomials in x with coefficients from the complex numbers, such as z2 + 2(1−i)z + (15 + 6i), or more generally from other fields and rings. See Fundamental Theorem of Algebra, polynomial ring.
- scheduling
- Schläfli, Ludwig
- Schläfli symbol
- Schrödinger, Erwin Rudolf Alexander
- Schrödinger's cat
- Schrödinger's equation
- Schur decomposition
- Schwartz distribution
- scientific notation
- sd
- SDE
- se
- seasonal variation
- secant
- secant
- secant method
- sech
- second
- second
- second derivative
- second derivative test
- second fundamental form
- second-order logic
- second-order partial derivative
- section