Incircle
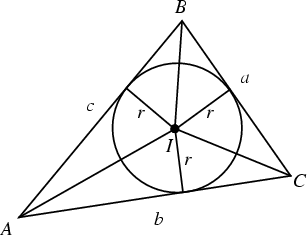
The Inscribed Circle of a Triangle 
. The center 
is called the Incenter and theRadius 
the Inradius. The points of intersection of the incircle with 
are the Vertices of the Pedal Triangle of with the Incenter as the Pedal Point (c.f. TangentialTriangle). This Triangle is called the Contact Triangle.
The Area 
of the Triangle is given by
 |  |  | |
|  |
where
is the Semiperimeter.Using the incircle of a Triangle as the Inversion Center, the sides of the Triangle and itsCircumcircle are carried into four equal Circles (Honsberger 1976, p. 21). Pedoe (1995, p. xiv) gives aGeometric Construction for the incircle.
See also Circumcircle, Congruent Incircles Point, Contact Triangle, Inradius, TriangleTransformation Principle
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 11-13, 1967. Honsberger, R. Mathematical Gems II. Washington, DC: Math. Assoc. Amer., 1976. Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 182-194, 1929. Pedoe, D. Circles: A Mathematical View, rev. ed. Washington, DC: Math. Assoc. Amer., 1995.
- Quindecillion
- Quintet
- Quintic Equation
- Quintic Surface
- Quintillion
- Quintuple
- Quintuple Product Identity
- Quintuplet
- Quota Rule
- Quota System
- Quotient
- Quotient-Difference Algorithm
- Quotient-Difference Table
- Quotient Group
- Quotient Ring
- Quotient Rule
- Quotient Space
- q-Vandermonde Sum
- R
- R-
- R+
- Raabe's Test
- Rabbit Constant
- Rabbit-Duck Illusion
- Rabbit Sequence