| 释义 |
Lyapunov Characteristic ExponentThe Lyapunov characteristic exponent [LCE] gives the rate of exponential divergence from perturbed initial conditions. To examinethe behavior of an orbit around a point 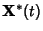 , perturb the system and write , perturb the system and write
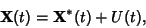 | (1) |
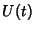 is the average deviation from the unperturbed trajectory at time is the average deviation from the unperturbed trajectory at time 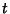 . In a Chaotic region, the LCE . In a Chaotic region, the LCE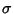 is independent of is independent of 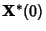 . It is given by the Osedelec Theorem, which states that . It is given by the Osedelec Theorem, which states that
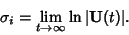 | (2) |
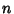 -dimensional mapping, the Lyapunov characteristic exponents are given by -dimensional mapping, the Lyapunov characteristic exponents are given by
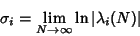 | (3) |
 , ..., , ..., 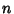 , where , where 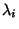 is the Lyapunov Characteristic Number. is the Lyapunov Characteristic Number.
One Lyapunov characteristic exponent is always 0, since there is never any divergence for a perturbed trajectory in the directionof the unperturbed trajectory. The larger the LCE, the greater the rate of exponential divergence and the wider thecorresponding Separatrix of the Chaotic region. For the Standard Map, an analytic estimate of thewidth of the Chaotic zone by Chirikov (1979) finds
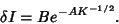 | (4) |
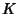 , some relationship likely exists connecting the two. Let a trajectory (expressed as a Map) have initial conditions , some relationship likely exists connecting the two. Let a trajectory (expressed as a Map) have initial conditions 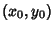 and a nearby trajectory have initial conditions and a nearby trajectory have initial conditions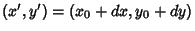 . The distance between trajectories at iteration . The distance between trajectories at iteration 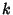 is then is then
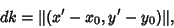 | (5) |
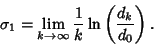 | (6) |
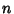 -dimensional phase space (Map), there are -dimensional phase space (Map), there are 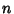 Lyapunov characteristic exponents Lyapunov characteristic exponents 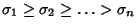 . However, because the largest exponent . However, because the largest exponent 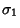 will dominate, this limit is practically usefulonly for finding the largest exponent. Numerically, since will dominate, this limit is practically usefulonly for finding the largest exponent. Numerically, since 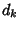 increases exponentially with increases exponentially with 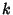 , after a few steps theperturbed trajectory is no longer nearby. It is therefore necessary to renormalize frequently every , after a few steps theperturbed trajectory is no longer nearby. It is therefore necessary to renormalize frequently every 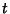 steps. Defining steps. Defining
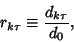 | (7) |
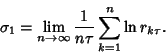 | (8) |
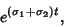 | (9) |
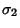 can be extracted if can be extracted if 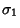 is known. The process may be repeated to find smaller exponents. is known. The process may be repeated to find smaller exponents.
For Hamiltonian Systems, the LCEs exist in additive inverse pairs, so if 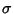 is an LCE, thenso is is an LCE, thenso is 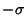 . One LCE is always 0. For a 1-D oscillator (with a 2-D phase space), the two LCEs therefore must be . One LCE is always 0. For a 1-D oscillator (with a 2-D phase space), the two LCEs therefore must be 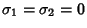 , so the motion is Quasiperiodic and cannot be Chaotic. Forhigher order Hamiltonian Systems, there are always at least two 0 LCEs, but other LCEs may enter inplus-and-minus pairs , so the motion is Quasiperiodic and cannot be Chaotic. Forhigher order Hamiltonian Systems, there are always at least two 0 LCEs, but other LCEs may enter inplus-and-minus pairs  and and 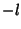 . If they, too, are both zero, the motion is integrable and not Chaotic. Ifthey are Nonzero, the Positive LCE . If they, too, are both zero, the motion is integrable and not Chaotic. Ifthey are Nonzero, the Positive LCE  results in an exponential separation of trajectories, which corresponds to aChaotic region. Notice that it is not possible to have all LCEs Negative, which explains why convergence oforbits is never observed in Hamiltonian Systems. results in an exponential separation of trajectories, which corresponds to aChaotic region. Notice that it is not possible to have all LCEs Negative, which explains why convergence oforbits is never observed in Hamiltonian Systems.
Now consider a dissipative system. For an arbitrary 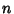 -D phase space, there must always be one LCE equal to 0, since a perturbationalong the path results in no divergence. The LCEs satisfy -D phase space, there must always be one LCE equal to 0, since a perturbationalong the path results in no divergence. The LCEs satisfy 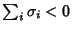 . Therefore, for a 2-D phase space of adissipative system, . Therefore, for a 2-D phase space of adissipative system, 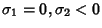 . For a 3-D phase space, there are three possibilities: . For a 3-D phase space, there are three possibilities: - 1. (Integrable):
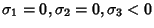 , , - 2. (Integrable):
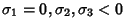 , , - 3. (Chaotic):
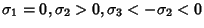 . .
See also Chaos, Hamiltonian System, Lyapunov Characteristic Number,Osedelec Theorem
References
Chirikov, B. V. ``A Universal Instability of Many-Dimensional Oscillator Systems.'' Phys. Rep. 52, 264-379, 1979. |
![]() , perturb the system and write
, perturb the system and write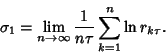
![]() is an LCE, thenso is
is an LCE, thenso is ![]() . One LCE is always 0. For a 1-D oscillator (with a 2-D phase space), the two LCEs therefore must be
. One LCE is always 0. For a 1-D oscillator (with a 2-D phase space), the two LCEs therefore must be ![]() , so the motion is Quasiperiodic and cannot be Chaotic. Forhigher order Hamiltonian Systems, there are always at least two 0 LCEs, but other LCEs may enter inplus-and-minus pairs
, so the motion is Quasiperiodic and cannot be Chaotic. Forhigher order Hamiltonian Systems, there are always at least two 0 LCEs, but other LCEs may enter inplus-and-minus pairs ![]() and
and ![]() . If they, too, are both zero, the motion is integrable and not Chaotic. Ifthey are Nonzero, the Positive LCE
. If they, too, are both zero, the motion is integrable and not Chaotic. Ifthey are Nonzero, the Positive LCE ![]() results in an exponential separation of trajectories, which corresponds to aChaotic region. Notice that it is not possible to have all LCEs Negative, which explains why convergence oforbits is never observed in Hamiltonian Systems.
results in an exponential separation of trajectories, which corresponds to aChaotic region. Notice that it is not possible to have all LCEs Negative, which explains why convergence oforbits is never observed in Hamiltonian Systems.![]() -D phase space, there must always be one LCE equal to 0, since a perturbationalong the path results in no divergence. The LCEs satisfy
-D phase space, there must always be one LCE equal to 0, since a perturbationalong the path results in no divergence. The LCEs satisfy ![]() . Therefore, for a 2-D phase space of adissipative system,
. Therefore, for a 2-D phase space of adissipative system, ![]() . For a 3-D phase space, there are three possibilities:
. For a 3-D phase space, there are three possibilities: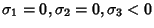 ,
,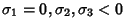 ,
,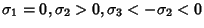 .
.