“Modulo Multiplication Group”的概念、定义、习题解题方法及翻译-数学辞典

单词

Modulo Multiplication Group

释义

Modulo Multiplication Group

A Finite Group of Residue Classes prime to under multiplication mod . isAbelian of Order , where is the Totient Function.The following table gives the modulo multiplication groups of small orders.

Group		Elements
	1	1
	2	1, 2
	2	1, 3
	4	1, 2, 3, 4
	2	1, 5
	6	1, 2, 3, 4, 5, 6
	4	1, 3, 5, 7
	6	1, 2, 4, 5, 7, 8
	4	1, 3, 7, 9
	10	1, 2, 3, 4, 5, 6, 7, 8, 9, 10
	4	1, 5, 7, 11
	12	1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11
	6	1, 3, 5, 9, 11, 13
	8	1, 2, 4, 7, 8, 11, 13, 14
	8	1, 3, 5, 7, 9, 11, 13, 15
	16	1, 2, 3, ..., 16
	6	1, 5, 7, 11, 13, 17
	18	1, 2, 3, ..., 18
	8	1, 3, 7, 9, 11, 13, 17, 19
	12	1, 2, 4, 5, 7, 8, 10, 11, 13, 16, 17, 19
	10	1, 3, 5, 7, 9, 13, 15, 17, 19, 21
	22	1, 2, 3, ..., 22
	8	1, 5, 7, 11, 13, 17, 19, 23

is a Cyclic Group (which occurs exactly when has a Primitive Root) Iff is of one of theforms , 4, , or , where is an Odd Prime and (Shanks 1993, p. 92).

Isomorphic modulo multiplication groups can be determined using a particular type of factorizationof as described by Shanks (1993, pp. 92-93). To perform this factorization (denoted ), factor in thestandard form

(1)

Now write the factorization of the Totient Function involving each power of an Odd Prime

(2)

(3)

where

(4)

denotes the explicit expansion of

(i.e.,

), and the last term is omitted if

.If

, write

(5)

Now combine terms from the odd and even primes. For example, consider

. The only odd prime factor is 13,so factoring gives

. The rule for the powers of 2 gives

. Combining these two gives

. Other explicit values of

are given below.

and are isomorphic Iff and are identical. More specifically, the abstract Groupcorresponding to a given can be determined explicitly in terms of a Direct Product ofCyclic Groups of the so-called Characteristic Factors, whose productis denoted . This representation is obtained from as the set of products of largest powers of each factor of. For example, for , the largest power of is and the largest power of 3 is , so thefirst characteristic factor is , leaving (i.e., only powers of two). The largest power remaining is, so the second Characteristic Factor is 2, leaving 2, which is the third and last Characteristic Factor. Therefore, , and the group is isomorphic to .

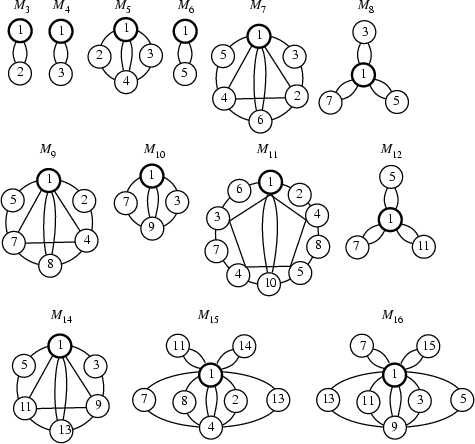
The following table summarizes the isomorphic modulo multiplication groups for the first few and identifies thecorresponding abstract Group. No is Isomorphic to , , or . However,every finite Abelian Group is isomorphic to a Subgroup of for infinitely many different values of (Shanks 1993, p. 96). Cycle Graphs corresponding to for small are illustrated above, and morecomplicated Cycle Graphs are illustrated by Shanks (1993, pp. 87-92).

Group	Isomorphic

	, ,
	,
	,
	, , ,
	, , ,

	,
	,
	, , ,
	,

	, ,
	, , ,
	,
	, ,
	,
	, , , , , ,
	,
	,
	,

The number of Characteristic Factors of for , 2, ... are 1, 1, 1, 1, 1, 1,1, 2, 1, 1, 1, 2, ... (Sloane's A046072). The number of Quadratic Residues in for aregiven by (Shanks 1993, p. 95). The first few for , 2, ... are 0, 1, 1, 1, 2, 1, 3, 1, 3, 2, 5, 1, 6,... (Sloane's A046073).

In the table below, is the A000010) factored into CharacteristicFactors, is the A011773), and are the smallestgenerators of the group (of which there is a number equal to the number of CharacteristicFactors).


3	2	2	2	27	18	18	2
4	2	2	3	28		6	13, 3
5	4	2	2	29	28	28	2
6	2	2	5	30		4	11, 7
7	6	6	3	31	30	30	3
8		2	7, 3	32		8	31, 3
9	6	6	2	33		10	10, 2
10	4	4	3	34	16	16	3
11	10	10	2	35		12	6, 2
12		2	5, 7	36		6	19,5
13	12	12	2	37	36	36	2
14	6	6	3	38	18	18	3
15		4	14, 2	39		12	38, 2
16		4	15, 3	40		4	39, 11, 3
17	16	16	3	41	40	40	6
18	6	6	5	42		6	13, 5
19	18	18	2	43	42	42	3
20		4	19, 3	44		10	43, 3
21		6	20, 2	45		12	44, 2
22	10	10	7	46	22	22	5
23	22	22	5	47	46	46	5
24		2	5, 7, 13	48		4	47, 7, 5
25	20	20	2	49	42	42	3
26	12	12	7	50	20	20	3

See also Characteristic Factor, Cycle Graph, Finite Group, Residue Class

References

Riesel, H. ``The Structure of the Group .'' Prime Numbers and Computer Methods for Factorization, 2nd ed. Boston, MA: Birkhäuser, pp. 270-272, 1994.

Shanks, D. Solved and Unsolved Problems in Number Theory, 4th ed. New York: Chelsea, pp. 61-62 and 92, 1993.

Sloane, N. J. A. Sequences A011773,A046072,A046073, andA000010/M0299in ``An On-Line Version of the Encyclopedia of Integer Sequences.''http://www.research.att.com/~njas/sequences/eisonline.html.

Weisstein, E. W. ``Groups.'' Mathematica notebook Groups.m.

随便看

数学辞典收录了8975条数学词条，基本涵盖了常用数学知识及数学英语单词词组的翻译及用法，是数学学习的有利工具。