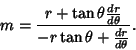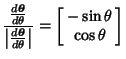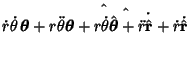| 释义 |
Polar CoordinatesThe polar coordinates  and and  are defined by are defined by
In terms of 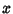 and and  , ,
The Arc Length of a polar curve given by  is is
 | (5) |
 | (6) |
 | (7) |
 is is
 | (8) |
 at the point at the point  is given by is given by
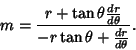 | (9) |
 is is
 | (10) |
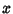 -axis if replacing -axis if replacing  by by 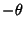 in its equation produces an equivalentequation, symmetric about the in its equation produces an equivalentequation, symmetric about the  -axis if replacing -axis if replacing  by by 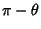 in its equation produces an equivalentequation, and symmetric about the origin if replacing in its equation produces an equivalentequation, and symmetric about the origin if replacing  by by  in its equation produces an equivalent equation. in its equation produces an equivalent equation.
In Cartesian coordinates, the Position Vector and its derivatives are
In polar coordinates, the Unit Vectors and their derivatives are
 |  |  | (15) |  |  |  | (16) |  |  | 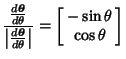 | (17) |  |  |  | (18) |  |  |  | (19) |  |  |  | |  |  | 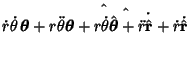 | | | |  |  | | | |  |  | | | |  |  | (20) |
See also Cardioid, Circle, Cissoid, Conchoid, Curvilinear Coordinates, CylindricalCoordinates, Equiangular Spiral, Lemniscate, Limaçon, Rose |
![]() and
and ![]() are defined by
are defined by